Optimizing Brillouin Optical Time-Domain Analyzers Based on Gain Spectrum Engineering

Summary

A protocol for Brillouin optical time-domain analyzers based on gain spectrum engineering is presented. Enhancements in the sensing performance, including sensing range and measurand resolution are achieved and the excess Brillouin intensity noise is studied. The protocol introduces a new way to enhance distributed Brillouin sensing performance.

Abstract

Demonstrated is a unique method for sensing performance enhancement in Brillouin optical time-domain analyzers (BOTDA). A Brillouin gain spectrum (BGS) is superimposed with two symmetric Brillouin loss spectra (BLS). This leads to a complex engineered spectrum shape that is more resistant to the sensing system noise. Instead of only one pump and probe interaction as in the conventional BOTDA setup, three optical probe waves are exploited, with one probe located in the BGS and the other two symmetrically in the BLS. Due to the resistance and insensitivity of the engineered spectrum shape to the noise, the sensing performance is enhanced by 60% and the measurand resolution is doubled.

Introduction

Distributed fiber sensing (DFS) is a unique mechanism that employs a whole fiber as a sensing medium. It has attracted a lot of interest due to the low fiber loss; small size; and the ability to be easily embedded in various structures, such as dams, bridges, and buildings, to perform environment surveillance as an artificial nerve system. In comparison to applying numerous traditional point sensors, such as fiber Bragg gratings (FBG), it provides a more efficient and cost-effective solution in a wide range of large-scale sensing tasks, such as infrastructure and structural health monitoring¹.

Current distributed sensors exploit different scattering mechanisms inside the fiber to measure temperature and strain distribution. Among them, DFS based on Brillouin scattering is the most attractive due to the striking advantages of the stimulated Brillouin scattering (SBS), such as high signal-to-noise ratio (SNR), low threshold, and the sensitivity to both temperature² and strain³. SBS can be classically described as an interaction between the incident optical continuous waves (CW), i.e., the pump, and the counter-propagating CW probe wave via an acoustic wave. According to the conservation of energy and momentum, the probe wave is frequency downshifted to the pump. This shift is called Brillouin frequency shift (BFS). Considering the finite lifetime of a 10 ns acoustic wave, there is a finite spectral distribution of the refracted wave, also called Brillouin gain spectrum (BGS), in which the BFS is the frequency difference between the pump wave and the peak center frequency. The interaction between the waves leads to a frequency down-shifted gain region and a frequency up-shifted loss region where the probe wave gets amplified and attenuated, respectively. For a standard single mode fiber (SSMF) in C-Band, the BFS is approximately 11 GHz and the BGS has a Lorentzian shape with an ultra-narrow full-width at half maximum (FWHM) of 10-30 MHz, which can be further reduced to 3.4 MHz with specific techniques^4,5,6,7. Based on these characteristics, SBS can also be applied in microwave photonics filters^8,9,10, optical filters¹¹, slow and fast light^12,13,14, and high resolution optical spectroscopy^7,15.

One of the most promising SBS applications is distributed Brillouin sensing. These sensors exploit the BFS dependence on temperature and strain. The first to be demonstrated was the Brillouin optical time-domain analyzer (BOTDA)¹⁶, which is the most consolidated time-domain distributed Brillouin sensing technique. It differs from the conventional CW-SBS interaction in that it exploits the SBS interaction between a pulsed pump wave and a probe CW so that the environmental information is locally interrogated on every fiber section. The pump or probe frequency is usually fixed while the probe or the pump frequency is scanned in the vicinity of the BFS. The probe power is recorded for BGS reconstruction and the BFS is ideally the peak frequency of the local BGS at each fiber section. However, due to the inevitable system noise, the BGS peak is usually ambiguous and a fitting algorithm has to be applied¹⁷, which leads to a certain estimation error in frequency¹⁸ and influences the measurand resolution.

Statistically, the BFS estimation error is inversely proportional to the system signal-to-noise ratio (SNR). The most straightforward way to enhance the SNR is to increase the pump and probe power. However, these are limited by modulation instability (MI)¹⁹ and non-local effects (NLE)^20,21 to ~20 dBm and -14 dBm, respectively. Numerous techniques, such as coding²² and Raman based inline-amplification²³ have been proposed to break these limits. Recently, it has been reported that this frequency error can be minimized by choosing a proper fitting algorithm²⁴. Relatedly, measurements exploiting the Brillouin phase and a linear fitting algorithm are also reported to have a reduced frequency error²⁵, which indicates the potential of a well-engineered BGS to sensing performance enhancement. Another option to enhance SNR is noise reduction. However, according to the traditional point of view, the sensing system noise comes mainly from the detector (i.e., common-mode noise, including dark noise, shot noise, etc.)^26,27 and improvement is less likely.

The basic idea of this paper is to engineer the BGS by the superposition of a conventional BGS with two symmetric Brillouin loss spectra (BLS) (see Figure 1). In comparison to a conventional BGS spectrum, which follows a Lorentzian shape, the engineered spectrum is sharper and more robust with the same level of system noise. Thus, the noise has less influence on the determination of the peak frequency. This can be verified by collecting and fitting the BGS measurement data a statistically significant number of times. Because of this better resistance to the noise, sensing performance enhancements are achieved, including the sensing range by 60% and doubled measurand resolution, i.e., a 50% reduced frequency error. Due to the involvement of the measurement with Brillouin loss interaction in part of the engineered BGS, a direct comparison of the trace noise with and without Brillouin interaction is made. Owing to the circumvention of the excess Brillouin noise, the trace with the engineered BGS is much clearer.

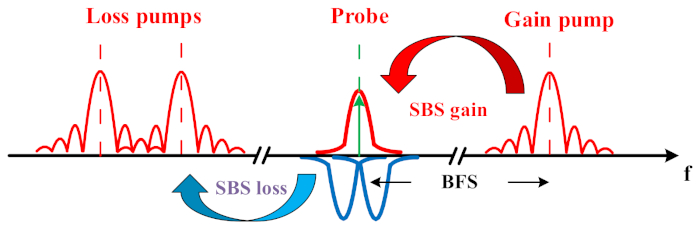
Figure 1: Schematic of an engineered BGS by the superposition of one Brillouin gain and two symmetric Brillouin loss spectra. Please click here to view a larger version of this figure.

Protocol

1. Selecting optimized parameters for the spectrum engineering via simulation

1. Model the engineered BGS g_SBS(ν,z) with the equations^28,29
   
   as implemented by, for example, the supplemental MATLAB script.
   NOTE: Here, G(ν) is the complex gain coefficient, calculated in the script as G_complex within the SBS_g function for the conventional BGS or SBS_gl function for the engineered BGS; g(ν,z) is the local complex Brillouin gain; and g_SBS and φ_SBS are the real and imaginary part of g(ν,z) and symbolize the BGS and SBS phase response at the location z from the pump launching end, respectively. In the script, g_SBS is symbolized by the variables SBS_g_log and SBS_gl_log for the conventional and engineered BGS in logarithmic scale, respectively. P_p = 20 dBm (P_pump in the script) is the pump pulse peak power at the fiber launching end; ν_B is the BFS (normalized in the script); g₀ = 0.2 W^-1m^-1 (g₀ in the script) is the Brillouin gain coefficient; Δν_B = 50 MHz (gamma_B in the script) is the FWHM of the BGS; α = 0.2 dB/km is the fiber loss coefficient and is represented by alpha_log and alpha_lin as the value in logarithmic and linear scale in the script, respectively; ν is the probe wave frequency so that the pump and probe frequency offset ν - ν_B is scanned from -250 MHz to 250 MHz. The pump and probe frequency offset is represented in the script as the global vector f. The term g₁ = mg₀ is the loss factor; 2d∙Δν_B is the frequency offset between the two losses; and L_eff = 10 m (L_eff in the script) is the Brillouin interaction length for 100 ns pulse width. The engineered spectral shape can be easily adjusted by tuning two normalized factors, m and d. The conventional BGS can be modelled simply by m = 0.
2. Add random noise (i.e., additive white Gaussian noise) with the same level both on the conventional and engineered BGS. Adjusting the parameter noise_level in the script can change the noise level.
3. Fit the conventional noisy BGS with the Lorentzian function (Lorentz_g_gain_fun function in the script) and the engineered noisy BGS with the superimposed Lorentzian function (Lorentz_gl_gain_fun function in the script) (see Figure 2A).
4. Determine the peak frequency offset due to the noise for the conventional and engineered BGS (Figure 2B).
5. Repeat steps 1.2-1.4 for N = 500 and collect all the peak frequency offsets Δf_ci and Δf_pi (represented by delta_g_g and delta_g_gl in the script, respectively) for the conventional and engineered BGS in the i^th process, respectively.

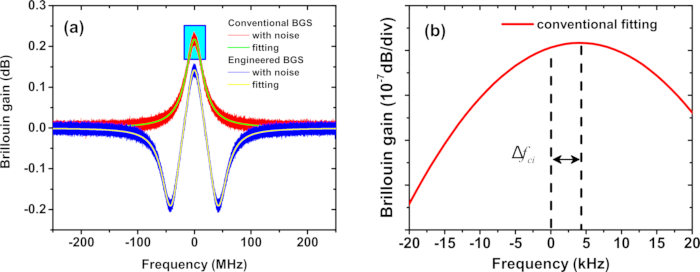
Figure 2: Simulated BGS. (A) Demonstration of the fitting of a typical Lorentzian (red) and engineered (blue) BGS in the simulation. (B) The Lorentzian BGS peak from (A). Δf_ci represents the BFS estimation error for the conventional BGS in the i^th measurement. Please click here to view a larger version of this figure.

6. Calculate the ratio of the standard deviation of the noise-induced BFS estimation errors as:
   
7. Plot η as a function of m and d (see Figure 3A). Find the minimum value η_min and the corresponding m and d. The advantage of the estimation accuracy will be maintained along the whole fiber²⁸.
8. Tune the value of z (i.e., the fiber length, also z in the script) in Equation (2) from 0 km to 60 km, step 50 m and repeat steps 1.1-1.7 with some selected m and d values. Then the ratio of the standard deviation as a function of the fiber length can be acquired (see Figure 3B).

2. Prepare and test the conventional BOTDA setup (highlighted block in Figure 4B)

1. Turn the laser diode (LD) on and check the functionality of the LD with the optical spectrum analyzer (OSA). Usually, a wavelength around 1,550 nm is used.
2. Connect the LD with the 10:90 optical coupler (OC). Check the power from the 10% OC output. If the power is higher than 13 dBm (the maximum input power of the polarization scrambler [Pol.S.]), lower the LD output power by lowering the LD current.
3. Connect the 10% OC output with the Pol.S. In order to avoid polarization fading¹, set the scrambling frequency to 1 kHz.
4. Check the power from the output of the Pol.S. If the power is higher than -3 dBm (the maximum input power limit of the semiconductor optical amplifier [SOA]), add attenuators until the power requirement is satisfied. Connect the Pol.S. with the SOA.
5. Apply the electrical pulse train signal with 100 ns pulse width and 4 kHz repetition rate from the pulse generator (PG) on the SOA. Ensure that the amplitude of the pulse signal is higher than the transistor-transistor logic (TTL) threshold of the SOA (i.e., 4 dBm) and the repetition rate satisfies the round-trip requirement³⁰.
6. Connect the output from the SOA with the Erbium-doped fiber amplifier (EDFA 1) and operate the EDFA in automatic current control (ACC) mode. Connect the output from EDFA 1 to port 1 of the circulator (Cir).
7. Check the optical pulse signal from port 2 of Cir in the digitizer by connecting it with the photodiode (PD). In order to avoid any damage to the PD, sufficient attenuation is necessary.
8. Calculate the optical pulse peak power by the waveform measured in the digitizer. The optical peak power P_o is estimated according to
   
   where V_E is the electrical pulse peak voltage, and R and R_f are the PD responsivity and transresistance. Take the protection attenuation into account in this calculation so that a correct input pump power into the fiber under test (FUT, 10.6 km) is evaluated.
9. Note the EDFA current value I₂₀ when the calculated optical pulse peak power reaches 20 dBm (MI threshold¹⁹). The extinction ratio (ER) of the optical pulse train can be evaluated according to the conservation of energy:
   
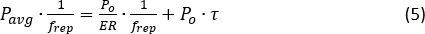
   where P_avg is the optical average power of the pulse train; f_rep is the repetition rate; and τ is the pulse width. The ER of the SOA is usually more than 30 dBm, efficiently avoiding NLE^31,32.
   NOTE: Switch off the EDFA before disconnecting the PD and continuing to build the system.
10. Connect port 2 of the Cir with one end of the FUT to complete setting up the pump branch. Check if the system will suffer from MI by checking the spectrum from the other end of the FUT in the OSA and setting the EDFA at a constant current value, I₂₀. If the detected spectrum is the same (in shape, not necessarily in amplitude) to the one measured in step 2.1, then the system is free from MI. Otherwise, when obvious spectrum broadening or peak splitting³³ are observed, reduce the EDFA 1 current to maintain the spectrum shape.
11. Connect the 90% output of the OC to a 50:50 OC 1 and connect one of the OC 1 outputs to the Mach-Zehnder modulator (MZM 1) via a polarization controller (PC). Set the polarization correctly, so that the output from MZM 1 is maximized (polarization alignment).
12. Apply the radio frequency (RF) signal from the RF generator (RFG 1) with the BFS of the FUT (for SSMF around 11 GHz) and 16 dBm amplitude to the MZM 1. Set the DC bias voltage of the MZM 1 so that the carrier is suppressed to the minimum.
13. Connect the output of the MZM 1 with fiber Bragg grating (FBG 1). Check the output spectrum from FBG 1 in the OSA so that the FBG 1 is set to block the upper frequency sideband and the carrier.
14. Connect the output from the FBG 1 to the EDFA 2, another 50:50 OC 2, and an isolator (ISO) consecutively.
15. Operate EDFA 2 also in ACC mode and set the current value to I_g, so that the output power from the ISO is lower than -14 dBm, minimizing NLE²⁰. Switch off the EDFA before disconnecting the power meter and continue the system building. Connect the ISO output to the other end of the FUT to complete the setup building of the gain probe branch.
16. Connect the Cir port 3 to the EDFA 4 (for pre-amplification³⁴) and PD. Set the EDFA to ACC mode. The current value I₄ should let the output power from EDFA be 4 smaller than the input limit of the PD. Connect the PD RF output to a 10 MHz RF low pass filter^35,36. Connect the filter output to the digitizer.
17. Connect the trigger of the digitizer with the synchronized (or inverse) output of the pulse generator. Set the digitizer parameters as follows: Sampling rate: 500 MSa/s; averaging: 4,096 times; number of samples: 10,000.
18. Set the current value of EDFA 1, EDFA 2, and EDFA 4 to I₂₀, I_g, and I₄, respectively. Run a trace recording program. Check the trace measured in the digitizer. If the trace amplitude follows the exponential decay, then the sensing system is MI free. Otherwise, reduce the current value of EDFA 1.

3. Measurement using the conventional BOTDA setup and data processing

1. Set the values of EDFA 1 and EDFA 2 to I₂₀ and I_g, respectively. Scan the frequency of RFG 1 in the range of BFS ± 90 MHz with 1 MHz steps. Record the trace from the recording program after each scanning step.
2. Calculate the local Brillouin gain by dividing the trace amplitude (in Brillouin interaction period) by each DC offset (non-Brillouin interaction period).
3. Retrieve the BGS at each fiber section by fitting the measured noisy BGS with the Lorentzian fitting. Determine the FWHM of the conventional BGS Δν_B0 from the fitting.
4. Repeat steps 3.1 and 3.2 for N = 48 times and collect all the estimated peak (BFS) frequency distributions along the fiber ν_Bci(z) in the i^th process (see Figure 5A).
5. Calculate the BFS estimation error as the standard deviation of the fitted BFS at each fiber section in the 48 measurements (see Figure 5B).

4. Preparing the rest of the setup

NOTE: In this case, m = 1 and d = 1.24 were used, per simulation results (see section 1 and Figure 3).

1. Connect the other output of the 50:50 OC 1 in the probe branch with the EDFA 3 and the 50:50 OC 3.
2. Connect one of the outputs of 50:50 OC 3 to a PC and MZM 2. Adjust the polarization so that the output from MZM 2 is maximized.
3. Apply the RF signal from RFG 2 with BFS - d∙Δν_B0 and 16 dBm amplitude on MZM 2. Check the modulation spectrum in the OSA and adjust the bias voltage to minimize the carrier amplitude.
4. Connect the output from MZM 2 to the input of an optical switch (OS 1), one of the inputs of 50:50 OC 4, and the FBG 2.
5. Check the optical signal in the OSA and adjust the FBG 2 center wavelength so that the carrier and the lower frequency sideband are blocked (see Figure 4A). The setup of the loss 1 probe branch will then be complete.
6. Connect the other output of the 50:50 OC 3 to a PC and MZM 3. Adjust the polarization so that the output from MZM 3 is maximized.
7. Apply the RF signal from the RFG 3 with BFS + d∙Δν_B0 and 16 dBm amplitude on MZM 3. Check the modulation spectrum in the OSA and adjust the bias voltage to minimize the carrier amplitude.
8. Connect the output from MZM 3 to a variable optical attenuator (VOA) with a switch function (OS 2) and the other input of 50:50 OC 4. Considering that the offset between the loss probe frequencies are relatively small in comparison to the FBG 2 transmission window (several GHz), the carrier and the lower frequency sideband from MZM 3 will also be blocked by FBG 2. The setup of the loss 2 probe branch will then be complete.
9. Close the OS 1, open the OS 2, and set the EDFA 3 current value to I_l so that the power of the loss 1 probe equals -14 dBm (m = 1).
10. Open the OS 1, close the OS 2, and adjust the attenuation of VOA so that the power of the loss 2 probe also equals -14 dBm.

5. Measurement using the complete proposed BOTDA setup and data processing

1. Close the OS 1, close the OS 2, and set the current value of EDFA 1, EDFA 2, EDFA 3, and EDFA 4 to I₂₀, I_g, I_l, and I₄, respectively. Scan the frequency of the RFG 1 in the range of BFS ± 90 MHz in 1 MHz steps. Frequencies from RFG 2 and RFG 3 scan correspondingly. Record the trace from the program after each scanning step.
2. Calculate the local Brillouin gain as in step 3.2 and retrieve the engineered BGS at each fiber section by fitting the measured noisy BGS with the superimposed Lorentzian function.
3. Repeat steps 5.1 and 5.2 for N = 48 times and collect all the estimated peak (BFS) frequency distributions along the fiber ν_Bpi(z) in the i^th process (see Figure 5A).
4. Calculate the BFS estimation error as the standard deviation of the fitted BFS at each fiber section in the 48 measurements (see Figure 5B).

Results

Figure 3 shows the simulation results. Points with η < 1 in Figure 3A indicate a smaller frequency error (higher measurand resolution) with the engineered BGS. The lower the value was, the bigger the advantage. The minimum ratio was at m = 1, indicating that a multiprobe instead of multipump scheme can be carried out (see Discussion). Figure 3B

Discussion

The most critical step during the experiment is the equalization of the three probe powers so that m = 1 and symmetry between the two Brillouin loss spectra is achieved. Besides the separate power check using the power meter at Cir port 2, as presented in steps 4.9 and 4.10, the power equalization can be more precisely checked in the digitizer. By setting the RF 1 frequency to ~11 GHz (the fiber BFS) and switching off EDFA 3, the conventional trace with the peak gain can be recorded in the digitizer (trace I). T...

Materials

Name	Company	Catalog Number	Comments
Current controller for laser diode	ILX Lightwave	LDX3220
Digitizer	Acqiris SA	U5309A-1039
Erbium doped fiber amplifier 1	Photop	PTEDFA-A-PA-C-SCH-15
Erbium doped fiber amplifier 2	LiComm	OFA-TCH
Erbium doped fiber amplifier 3	Calmar Optcom	AMP-ST30
Erbium doped fiber amplifier 4	Photop	PTEDFA-A-PA-C-SCH-15
Fiber Bragg grating 1	Advanced Optics Solutions	T-FBG
Fiber Bragg grating 2	Advanced Optics Solutions	T-FBG
Fiber under test	ofs
Isolator	General Photonics	S-15-NTSS
Laser diode	3SP Group	A1905 LMI
Mach-Zehnder modulator 1	Avanex	IM10
Mach-Zehnder modulator 2	Avanex	IM10
Mach-Zehnder modulator 3	Avanex	IM10
Nanosecond driving board for semiconductor optical amplifier	Highland Technology	T160-9 (28A160-9C)
Optical coupler 10:90	Newport		Benchtop coupler/WDM
Optical coupler 50:50	Newport		Benchtop coupler/WDM
Optical spectrum analyzer	Hewlett Packard	86145A
Optical switch 1	JDSU	SN12-1075NC
Photodiode	Thorlabs	D400FC
Polarization scrambler	General Photonics	PSY-101
Pulase generator	Hewlett Packard	8082A
Radio function generator 1	Anritsu	MG3692C
Radio function generator 2	Agilent Technology	E8257D
Radio function generator 3	HTM	T2100
Semiconductor optical amplifier	Thorlabs	SOA1013SXS
Temperature controller for laser diode	ILX Lightwave	LDT5948
Temperature controller for semiconductor optical amplifier	Tektronix	TED200
Variable optical attenuator	JDSU	mVOA-A1	With optical switch function