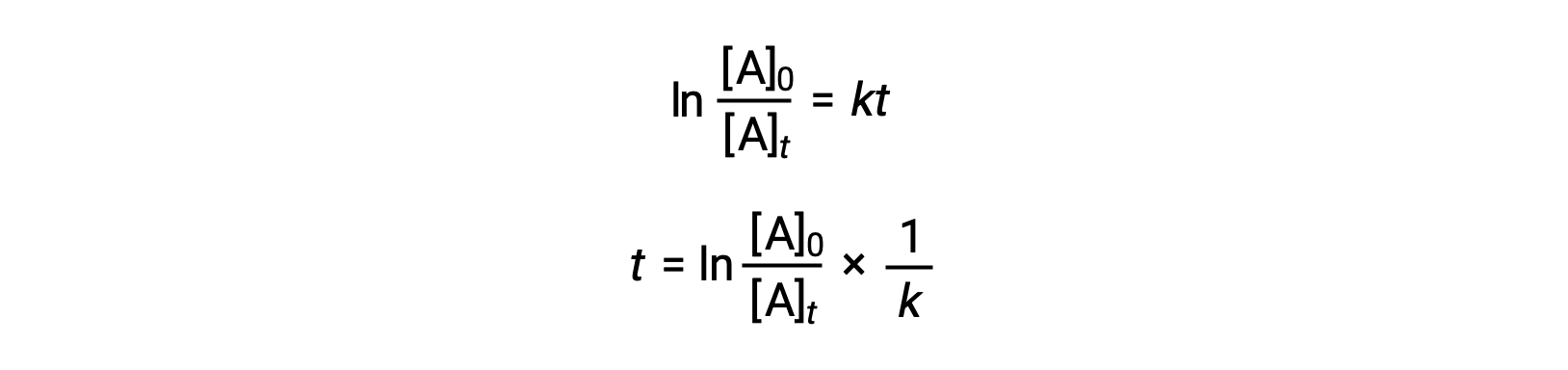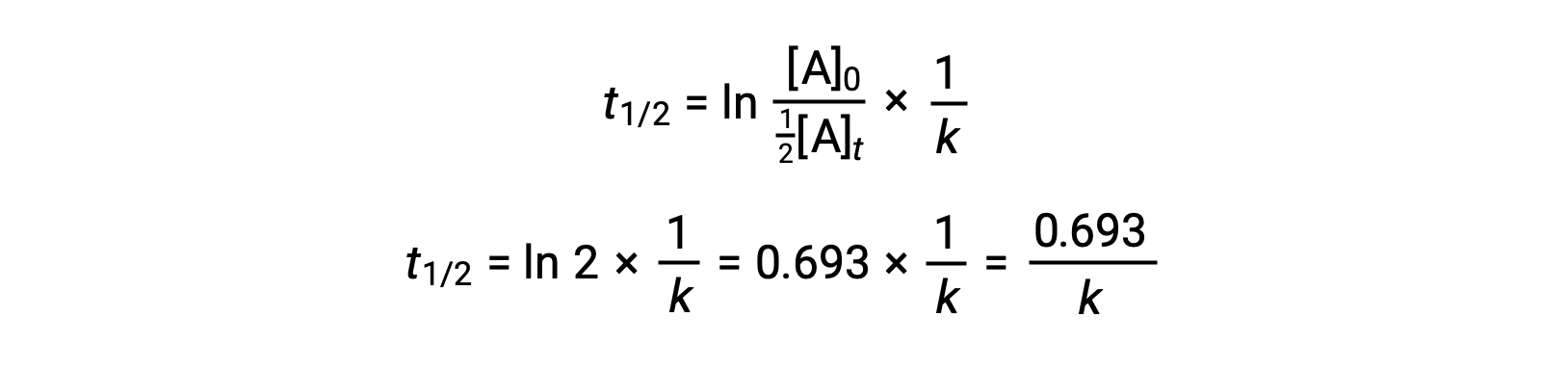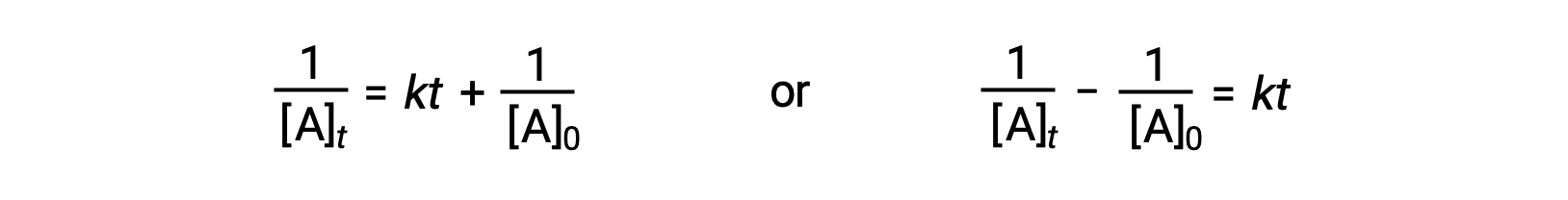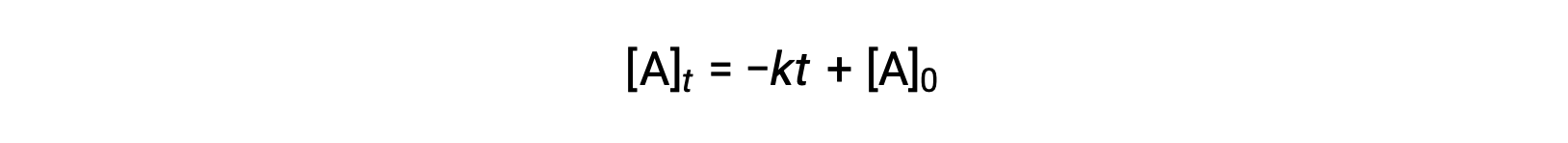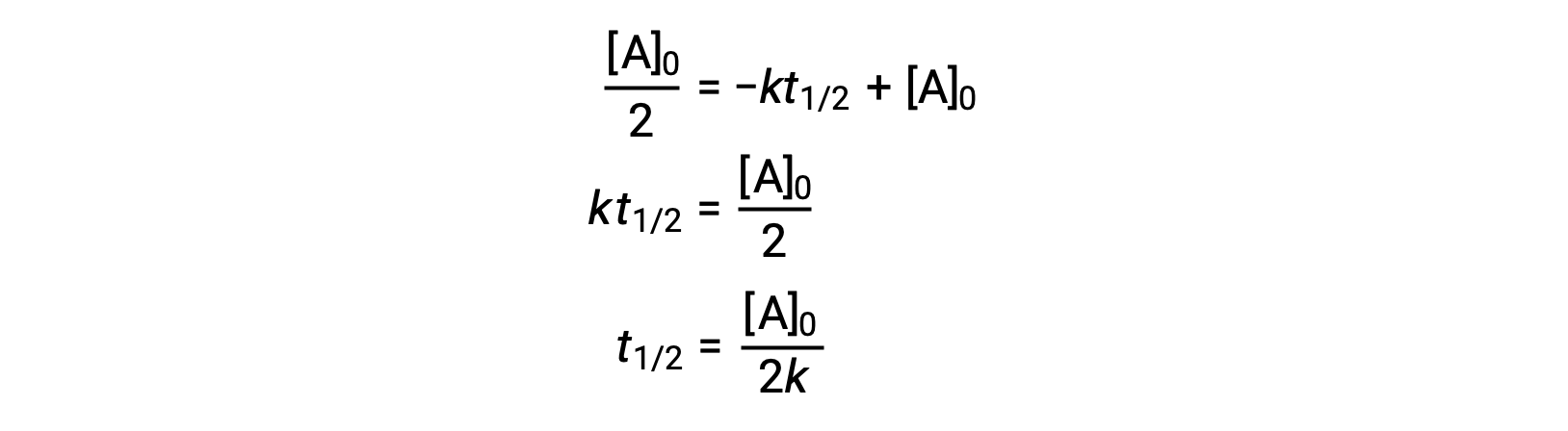During a chemical reaction, the reactant’s concentration decreases over time. The time taken to reduce it to half of its initial quantity is called the half-life of the reaction, abbreviated as t1/2.
Consider the refrigerant trichlorofluoromethane, which depletes the ozone layer. Its atmospheric residence time is 45 years and it is banned in many countries. But how long does it take for the concentration to drop to 50%? To estimate a reactant’s concentration after an elapsed time, the half-life can be used.
The half-life differs for each reaction type. Depending on the reaction order, a general half-life expression can be derived from the integrated rate laws.
Consider the zero-order integrated rate law. At t1/2, the reactant concentration is half of its initial concentration. On substitution of these parameters, an expression for the half-life is formulated.
The formula shows that the half-life for a zero-order reaction is dependent on and directly proportional to the initial reactant concentration. As the reactant concentration declines, the half-life continues to get shorter.
For a first-order reaction, the integrated rate expression is modified by substituting the time as t1/2 and the reactant quantity as half of its initial concentration. On solving, the expression for t1/2 is determined to be a constant.
Thus, the half-life of a first-order reaction is derived to be independent of the initial reactant concentration. Regardless of the decrease in the reactant concentration, the half-life remains constant.
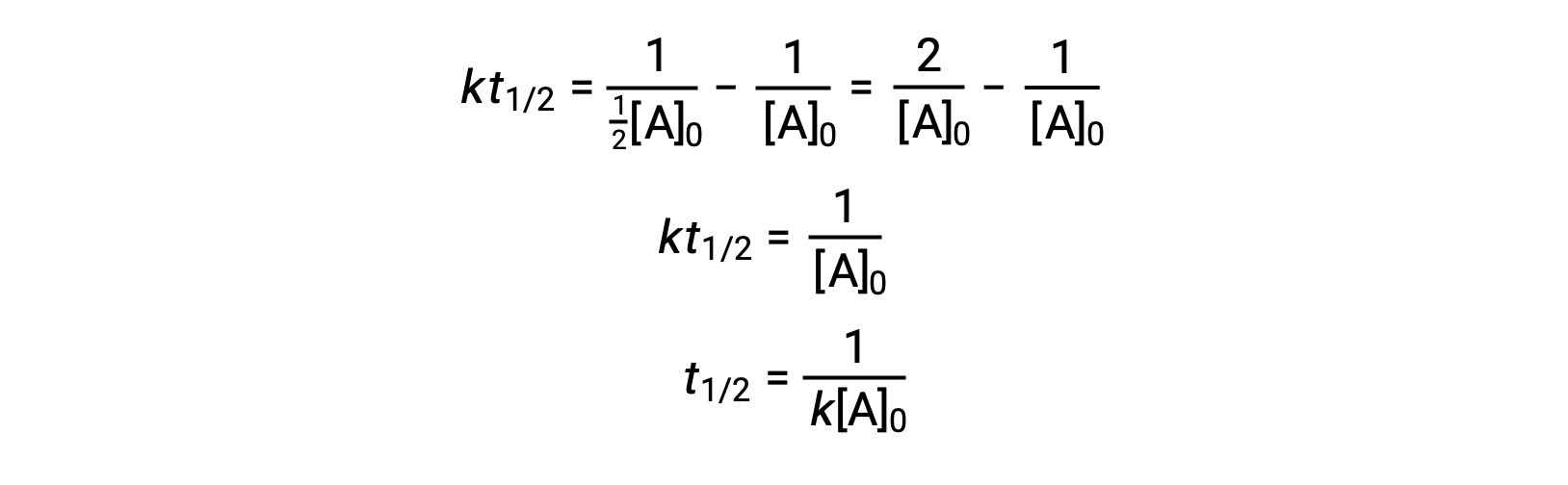
For a second-order reaction, the integrated rate expression is converted to t1/2 by substituting the time and reactant concentration parameters.
The half-lives of second-order reactions are inversely dependent on the initial reactant concentration. As the reactant concentration decreases, the t1/2 value increases; meaning, the half-life continues to get longer.
The half-life also helps to estimate the magnitude of the rate constant. It offers a relative comparison between different reaction rates; the shorter the half-life, the faster the reaction rate and vice-versa.
For instance, the radioactive isotope sodium-24, with a short half-life of 14.7 hours, exhibits a faster decay rate than cobalt-60, with a longer half-life of 5.3 years