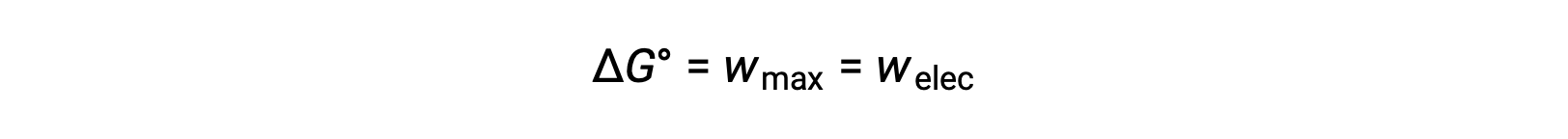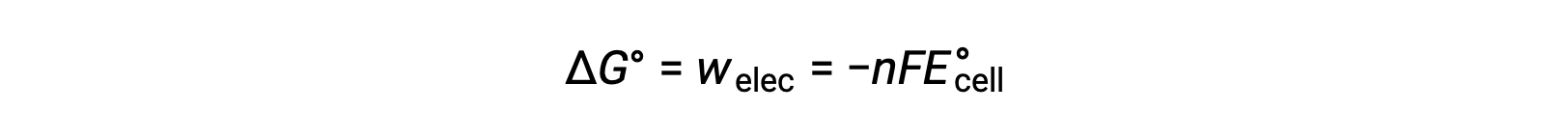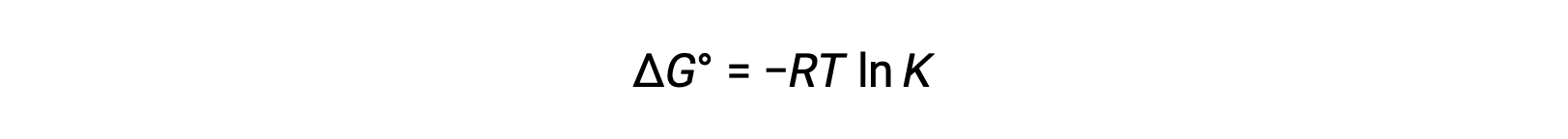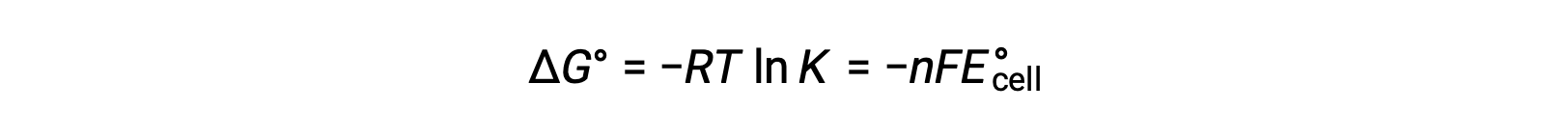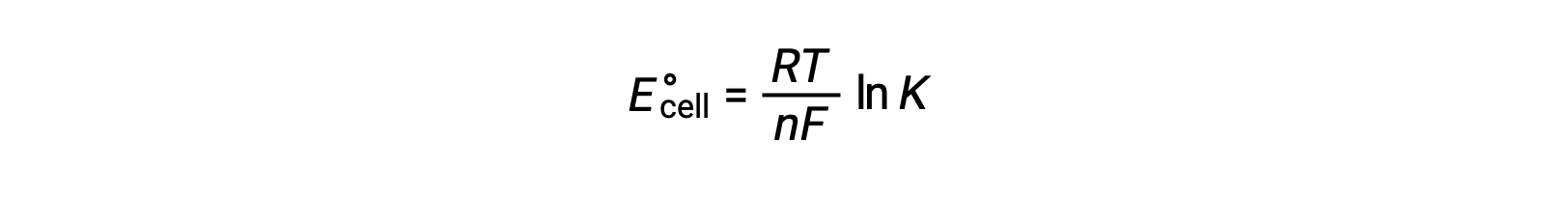The standard cell potential indicates a redox reaction’s spontaneity, so does the change in standard Gibbs free energy for a reaction. As both terms are a measure of reaction spontaneity, are they related to each other?

In a zinc-copper galvanic cell, the cell potential of 1.10 volts causes an electron flow, which is the maximum electrical work done by the cell. w_max is measured in joules and is expressed as the product of the total charge transferred in coulombs and the cell potential in volts.

The total charge, q, depends on n, the number of moles of electrons transferred during the reaction. In the zinc-copper galvanic cell, 2 moles of electrons are transferred from zinc to copper, so n = 2. To get the total charge, N is multiplied by Faraday’s constant, which is the magnitude of electric charge present in 1 mole of electrons: 96,485 coulombs.

Thus, the maximum electrical work performed by the zinc-copper galvanic cell is determined from the moles of electrons, Faraday’s constant, and the cell potential.

Here, all the energy for the electrical work is supplied by the cell itself, resulting in the system performing work on the surroundings which is denoted by a negative sign. 

Recall that Gibbs free energy is associated with a reaction’s energy available to perform work. Under standard-state conditions, the change in Gibbs free energy is a measure of the highest amount of work generated in a reaction.

Thus, the maximum work can be substituted with ΔG, allowing the free energy change of an electrochemical reaction to be determined.  

For the zinc-copper reaction, ΔG is −212 kilojoules, indicating that it is spontaneous. In comparison, a nickel-manganese redox reaction with a standard cell potential of −0.93 volts yields a value of +179 kilojoules, indicating that it is nonspontaneous.

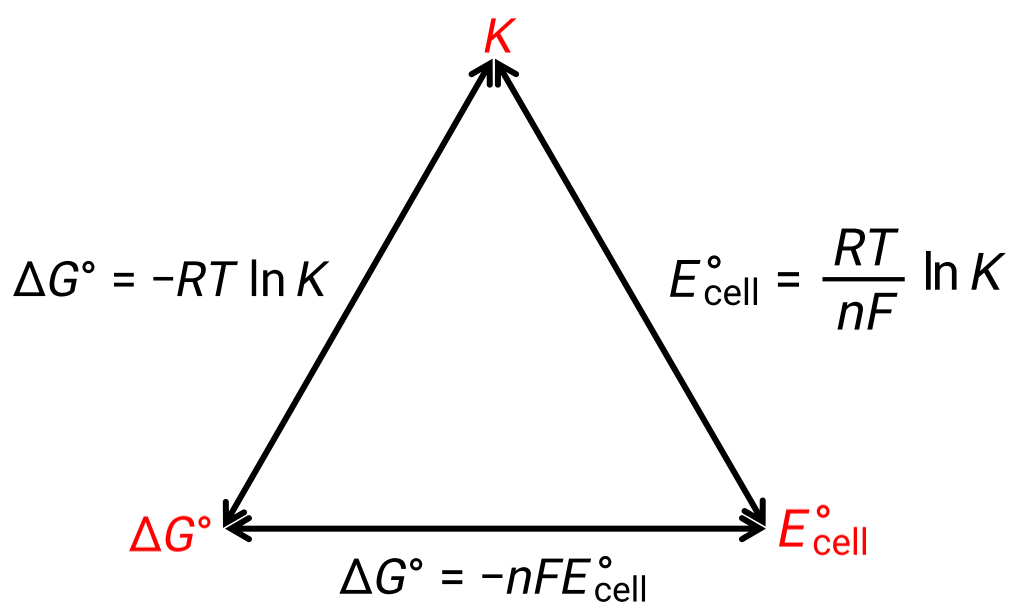
The standard free energy change is also related to the equilibrium constant, K. A large equilibrium constant indicates that the reaction lies to the product side correlating with a negative ΔG value, and vice versa. 

Given their relation to ΔG, the standard cell potential and equilibrium constant are also related. This relationship is derived by solving this equation for the cell potential and substituting ΔG with the gas constant, temperature, and the natural log of K.