3.8 : Kinematic Equations - II
The second kinematic equation expresses the final position of an object in terms of its initial position, the distance traveled with the initial constant velocity, and the distance traveled due to a change in velocity. Similar to the first kinematic equation, this equation is also only valid when the acceleration is constant throughout the motion of an object.
Suppose a car merges into freeway traffic on a 200 m long ramp. If its initial velocity is 10 m/s and it accelerates at 2 m/s2, then the time taken by the car to travel the 200 m long ramp can be calculated using the second kinematic equation. Here, the known quantities are the distance of 200 m, the initial velocity of the car of 10 m/s, and the acceleration of 2 m/s2. Using the second kinematic equation
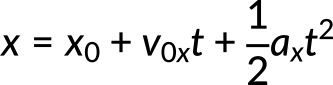
and substituting the known quantities in the above equation, we get
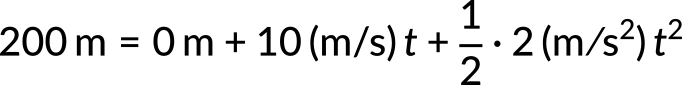
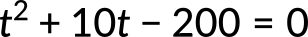
Using the quadratic formula to solve for time yields two solutions: t = 10 s and t = −20 s. A negative value for time is unreasonable, since that would mean the event happened 20 s before the motion began, therefore we can discard that solution. Thus, the time taken by the car to travel the 200 m ramp is 10 s.
This text is adapted from Openstax, University Physics Volume 1, Section 3.4: Motion with Constant Acceleration.
From Chapter 3:

Now Playing
3.8 : Kinematic Equations - II
Motion Along a Straight Line
9.3K Views

3.1 : Position and Displacement
Motion Along a Straight Line
17.2K Views

3.2 : Average Velocity
Motion Along a Straight Line
18.0K Views

3.3 : Instantaneous Velocity - I
Motion Along a Straight Line
12.3K Views

3.4 : Instantaneous Velocity - II
Motion Along a Straight Line
9.1K Views

3.5 : Average Acceleration
Motion Along a Straight Line
9.4K Views

3.6 : Instantaneous Acceleration
Motion Along a Straight Line
7.6K Views

3.7 : Kinematic Equations - I
Motion Along a Straight Line
10.3K Views

3.9 : Kinematic Equations - III
Motion Along a Straight Line
7.4K Views

3.10 : Kinematic Equations: Problem Solving
Motion Along a Straight Line
11.8K Views

3.11 : Free-falling Bodies: Introduction
Motion Along a Straight Line
8.0K Views

3.12 : Free-falling Bodies: Example
Motion Along a Straight Line
15.7K Views

3.13 : Velocity and Position by Graphical Method
Motion Along a Straight Line
7.2K Views

3.14 : Velocity and Position by Integral Method
Motion Along a Straight Line
5.9K Views
Copyright © 2025 MyJoVE Corporation. All rights reserved