10.6 : Relacionando magnitudes angulares y lineales - II
In the case of circular motion, the linear tangential speed of a particle at a radius from the axis of rotation is related to the angular velocity by the relation:
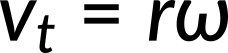
This could also apply to points on a rigid body rotating about a fixed axis. In a circular motion, both uniform and nonuniform, there exists a centripetal acceleration. The centripetal acceleration vector points inward from the particle executing circular motion toward the axis of rotation. In uniform circular motion, when the angular velocity is constant and the angular acceleration is zero, we observe a linear acceleration—that is, centripetal acceleration—since the tangential speed is constant. If the circular motion is nonuniform, then the rotating system has an angular acceleration, and we have both a linear centripetal acceleration and linear tangential acceleration.
The centripetal acceleration is due to a change in the direction of tangential velocity, whereas the tangential acceleration is due to any change in the magnitude of the tangential velocity. The tangential and centripetal acceleration vectors are always perpendicular to each other. To complete this description, a total linear acceleration vector is assigned to a point on a rotating rigid body or a particle executing circular motion at a radius r from a fixed axis. The total linear acceleration vector is the vector sum of the centripetal and tangential accelerations. The total linear acceleration vector in the case of nonuniform circular motion points at an angle between the centripetal and tangential acceleration vectors.
This text is adapted from Openstax, University Physics Volume 1, Section 10.3: Relating Angular and Translational Quantities.
Del capítulo 10:

Now Playing
10.6 : Relacionando magnitudes angulares y lineales - II
Rotación y cuerpos rígidos
5.4K Vistas

10.1 : Velocidad angular y desplazamiento
Rotación y cuerpos rígidos
15.1K Vistas

10.2 : Velocidad angular y aceleración
Rotación y cuerpos rígidos
9.1K Vistas

10.3 : Rotación con aceleración angular constante - I
Rotación y cuerpos rígidos
6.7K Vistas

10.4 : Rotación con aceleración angular constante - II
Rotación y cuerpos rígidos
5.9K Vistas

10.5 : Relacionando magnitudes angulares y lineales - I
Rotación y cuerpos rígidos
6.5K Vistas

10.7 : Momento de inercia
Rotación y cuerpos rígidos
12.0K Vistas

10.8 : Momento de inercia y energía cinética rotacional
Rotación y cuerpos rígidos
7.3K Vistas

10.9 : Momento de inercia: cálculos
Rotación y cuerpos rígidos
6.7K Vistas

10.10 : Momentos de inercia de objetos compuestos
Rotación y cuerpos rígidos
6.1K Vistas

10.11 : Teorema de los ejes paralelos
Rotación y cuerpos rígidos
6.5K Vistas

10.12 : Teorema del eje perpendicular
Rotación y cuerpos rígidos
2.7K Vistas

10.13 : Transformación vectorial en sistemas de coordenadas rotatorias
Rotación y cuerpos rígidos
1.5K Vistas

10.14 : Fuerza de Coriolis
Rotación y cuerpos rígidos
3.2K Vistas
ACERCA DE JoVE
Copyright © 2025 MyJoVE Corporation. Todos los derechos reservados