Convergent Polishing: A Simple, Rapid, Full Aperture Polishing Process of High Quality Optical Flats & Spheres

Podsumowanie

A novel optical polishing process, called “Convergent Polishing”, which enables faster, lower cost polishing, is described. Unlike conventional polishing processes, Convergent Polishing allows a glass workpiece to be polished in a single iteration and with high surface quality to its final surface figure without requiring changes to polishing parameters.

Streszczenie

Convergent Polishing is a novel polishing system and method for finishing flat and spherical glass optics in which a workpiece, independent of its initial shape (i.e., surface figure), will converge to final surface figure with excellent surface quality under a fixed, unchanging set of polishing parameters in a single polishing iteration. In contrast, conventional full aperture polishing methods require multiple, often long, iterative cycles involving polishing, metrology and process changes to achieve the desired surface figure. The Convergent Polishing process is based on the concept of workpiece-lap height mismatch resulting in pressure differential that decreases with removal and results in the workpiece converging to the shape of the lap. The successful implementation of the Convergent Polishing process is a result of the combination of a number of technologies to remove all sources of non-uniform spatial material removal (except for workpiece-lap mismatch) for surface figure convergence and to reduce the number of rogue particles in the system for low scratch densities and low roughness. The Convergent Polishing process has been demonstrated for the fabrication of both flats and spheres of various shapes, sizes, and aspect ratios on various glass materials. The practical impact is that high quality optical components can be fabricated more rapidly, more repeatedly, with less metrology, and with less labor, resulting in lower unit costs. In this study, the Convergent Polishing protocol is specifically described for fabricating 26.5 cm square fused silica flats from a fine ground surface to a polished ~λ/2 surface figure after polishing 4 hr per surface on a 81 cm diameter polisher.

Wprowadzenie

The major steps in a typical optical fabrication process include shaping, grinding, full aperture polishing, and sometimes small tool polishing^1-3. With increasing demand for high quality optical components for imaging and laser systems, there have been significant advancements in optical fabrication over the last several decades. For example, precision, deterministic material removal is now possible during the shaping and grinding processes with advancements in Computer Numerical Controlled (CNC) glass shaping machines. Similarly, small tool polishing technologies (e.g., computer controlled optical surfacing (CCOS), ion figuring, and magneto-rheological finishing (MRF)) have led to deterministic material removal and surface figure control, thus strongly impacting the optical fabrication industry. However, the intermediate step of the finishing process, full aperture polishing, still lacks high determinism, typically requiring skilled opticians to carry out multiple, often long, iterative cycles with multiple process changes to achieve to the desired surface figure^1-3.

The large number of polishing methods, process variables, and the complex chemical and mechanical interactions between the workpiece, lap and slurry^3-4 have made it challenging to transform optical polishing from an ‘art’ to a science. To achieve deterministic full aperture polishing, the material removal rate must be well understood. Historically, material removal rate has been described by the widely used Preston equation⁵

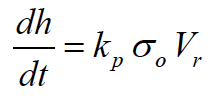 (1)

where dh/dt is the average thickness removal rate, k_p is the Preston constant, σ_o is the applied pressure, and V_r is the average relative velocity between the workpiece and the lap. Figure 1 schematically depicts the physical concepts that influence material removal rate as described the Preston Equation, including spatial and temporal variations in velocity and pressure, differences between the applied pressure and the pressure distribution that the workpiece experiences, and friction effects^6-8. In particular, the actual pressure distribution experienced by the workpiece is governed by a number of phenomena (described in detail elsewhere^6-8) which strongly affect resulting surface figure of the workpiece. Also, in the Preston Equation, the microscopic and molecular level effects are largely folded into the macroscopic Preston constant (k_p), which influences the overall material removal rate, micro-roughness, and even scratching on the workpiece. Various studies have expanded Preston’s model to account for microscopic slurry particle-pad-workpiece interactions to explain material removal rate and microroughness^9-16.

To achieve deterministic control of surface figure during full aperture polishing, each of the phenomena described above needs to be understood, quantified and then controlled. The strategy behind Convergent Polishing is to eliminate or minimize the undesirable causes of non-uniform material removal, either through engineered polisher design or by process control, such that removal is driven only by the workpiece-lap mismatch due to workpiece shape^7,17-18. Figure 2 illustrates how workpiece shape can lead to convergence based on the workpiece-lap mismatch concept. Consider a flat lap and a hypothetical workpiece of complex shape shown at the top left. The interface height mismatch (referred to as the gap, Δh_oL) influences the interface pressure distribution (σ) as:

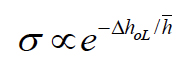 (2)

where h̅ is a constant describing the rate at which the pressure declines with an increase in gap Δh_oL⁶. In this example, the workpiece has the highest local pressure in the center (see bottom left of Figure 2), and hence this location will observe the highest initial material removal rate during polishing. As material is removed, the pressure differential across the workpiece will decrease due to a decrease in the workpiece-lap mismatch, and the workpiece will converge to the shape of the lap. At convergence, the workpiece pressure distribution, and hence material removal, will be uniform across the workpiece (see right side of Figure 2). This example is illustrated for a flat lap, however, the same concept applies for a spherical lap (either concave or convex). Again, this convergence process only works if all the other phenomena affecting spatial material non-uniformity have been eliminated. The specific procedural and engineering mitigations implemented in the Convergent Polishing protocol are described in the Discussion.

The protocol described in the following study is the Convergent Polishing process specifically for a 26.5 cm square fused silica glass workpiece starting from a fine ground surface. In 8 hours of polishing (4 hours/surface), this workpiece can achieve a polished flatness of ~λ/2 with very high surface quality (i.e., low scratch density).

Protokół

1. Preparation of Polisher and Slurry

First prepare the Convergent Polishing System (specifically called Convergent, Initial Surface Independent, Single Iteration, Rogue Particle-Free Polisher or CISR (pronounced ‘scissor’))^7,17 by installing the pad & septum, conditioning the pad, diluting & chemically stabilizing the slurry, and incorporating the slurry within the filtration system.

1. On the CISR polisher, adhere a polyurethane pad onto the granite lap base. Adhere pad one edge first and apply pressure in the direction towards the opposing edge to minimize air gaps. Trim the overhanging pad and then use a razor blade and roller to puncture and remove air bubbles if necessary.
2. For first time use and after every ~100 hr of polishing, diamond condition the pad using a CMP diamond conditioner (50 mm diameter; 0.6 psi applied pressure; 5 min dwell at each radial lap location with 25 mm spacing increments; lap rotation 25 rpm) with flowing DI water.
3. Between polishing iterations, remove any residual slurry and glass products from the pad using an in-situ ultrasonic cleaner (~2 min dwell at each radial location; lap rotation 5 rpm) with flowing DI water.
4. On the uniquely shaped septum weight, adhere double sided foam tape and then the septum material (e.g., pre-cut glass or other non-wearing material). Trim any overhanging foam tape to match the shape of the septum material and weight. Note the septum design (both shape and weight) changes for different sizes of the workpiece and lap^7,17.
5. Prepare polishing slurry to Baume 4 concentration (specifically mix by volume ~1 part cerium oxide polishing slurry and ~9 parts deionized (DI) water in a 11 L bucket). Check Baume using a Baume float. Add ~5 ml of KOH (10 M) to adjust pH to 9.5 and add ~120 ml (1 vol%) of proprietary surfactant¹⁹. Readjust pH and Baume every 24 hr of polishing.
6. Install bucket with the prepared slurry into filtration system. Then install the desired CMP particle filters into filtration system. Let slurry recirculate within filtration system for several hours.
7. Measure particle size distribution of slurry (e.g., using single particle optical sensing techniques) to ensure the tail end of the distribution is adequately rogue particle-free^9,20.

2. Preparation of Workpiece (Etching and Blocking)

Before polishing, chemically etch the as-received fine ground workpiece to reduce the amount of material removal needed to remove the sub-surface grinding damage²¹. Then, block the workpiece (if the aspect ratio (i.e., length/thickness) is >10) using a novel pitch button blocking (PBB) technique to prevent the workpiece from bending during blocking and polishing²².

1. Etch the fine ground workpiece (specifically a 265 x 265 x 8 mm³ fused silica glass flat) in a tank filled with HF:NH₄F (6:1 buffered oxide etch (BOE) 3x diluted with DI water) for 6 hr removing 10 µm of glass from surface of workpiece. CAUTION! BOE is extremely hazardous; wear appropriate personal protective equipment (PPE) . Remove workpiece from etch tank and aggressively rinse the workpiece with DI water and allow the workpiece to air dry vertically.
2. Inspect the workpiece for deep damage during the grinding process using a bright light inspection in a dark room. If no deep damage is found, proceed to the next step, else send workpiece back for re-grinding.
3. Heat up blocking pitch in a glue gun to ~95 ºC and place droplets (also called buttons) of pitch (~0.06 g) onto the face of the blocking plate in a 9 x 9 array (81 buttons with 26 mm spacing). For different size workpieces, refer to design rules for the ideal number, size, and spacings of pitch button²². Place the blocking plate with the applied buttons face up in a pre-heated oven at 70 ºC.
4. Apply tape to the face of the workpiece that is not to be polished. Avoid generating air bubbles or excessively stretching the tape.
5. Place the workpiece with tape side face down onto buttons on the blocking plate in the oven. Cover the workpiece-button-block to minimize convective flow. After 1.5 hr, set oven to cool 10 ºC/hr to room temperature. After cooling, the pitch thickness on the blocked workpiece should be ~1 mm.

3. Convergent Polishing

1. Turn on humidity system in environmental chamber of CISR polisher to prevent slurry from drying during polishing and to minimize rogue particles scratching the workpiece.
2. Install and mount the specifically designed and prepared septum into polisher. Install PBB workpiece into CISR polisher and lower boom to hold the workpiece.
3. Polish workpiece on CISR for 4 hr at a matched lap and workpiece rotation rate of 25 rpm with a radial stroke of ~75 mm and with a slurry flow from filtration system of 1 gal/min.
   NOTE: The blocking plate also serves as the loading weight on the workpiece corresponding to 0.6 psi applied pressure.
4. Turn off lap and workpiece rotation and slurry flow. Remove PBB workpiece from CISR polisher and submerse it into DI water filled bath. Wipe workpiece surface with cleanroom cloth while submerged. Remove PBB workpiece from bath and spray rinse with DI water.
5. Deblock workpiece by inserting a shim at workpiece-block interface. Remove tape from workpiece surface. Aggressively rinse workpiece with DI water and air dry.
6. PBB the opposing face of the workpiece as described in Section 2. Then repeat polishing procedure as described in Section 3.

4. Metrology and Inspection

1. Measure reflected wavefront (i.e., surface figure) of both sides of workpiece as well as transmitted wavefront using an interferometer.
2. Mount workpiece on bright light inspection station and measure the scratch/dig properties using optical fabrication standard methods. A short BOE etch of the workpiece, as described in step 2.1, can be used to expose hidden scratches for workpieces used in high fluence laser applications. For measurement of fine scratches or roughness on the workpiece, standard optical microscopy or white light interferometry may be used.
3. Store completed workpiece in a container minimizing contact with face of the workpiece.

Wyniki

The Convergent Polishing protocol described above allows a ground fused silica workpiece (in this case a 26.5 cm square) to be polished, in a single iteration of 4 hr per surface, to a peak-to-valley flatness of ~λ/2 (~330 nm) for low aspect ratio workpieces and ~1λ (~633 nm) for high aspect ratio workpieces (see Figure 3). Again, this process repeatedly converges workpieces to the same final surface figure without requiring changes to polishing parameters and is independent of the initial surf...

Dyskusje

As discussed in the Introduction, successful implementation of Convergent Polishing with respect to surface figure involves eliminating or minimizing all the phenomena that influence spatial material non-uniformity except that of workpiece-lap mismatch due to workpiece shape. If any one of these phenomena is not appropriately mitigated, either through process control or through appropriate engineering of the polisher, then the desired convergence point cannot be achieved or maintained; hence essentially every mitigation ...

Materiały

Name	Company	Catalog Number	Comments
MHN 50 mil Polyurethane Pad	Eminess Technologies	PF-MHN15A050L-56
Cerium oxide polishing slurry	Universal Photonics	HASTILITE PO
Septum Glass (waterjet cut)	Borofloat ; Schott	NA
Diamond conditioner	Morgan Advanced Ceramics	CMP-25035-SFT
Ultrasonic Cleaner	Advanced Sonics Processing System	URC4
Purification Optima Filter cartridge	3M	CMP560P10FC
Blocking Pitch	Universal Photonics	BP1
Blocking Tape	3M	#4712
Cleanroom Cloth	ITW Texwipe	AlphaWipe TX1013
Single Particle Optical Sensing	Paritcle Sizing Systems	Accusizer 780 AD