Following the work of Ernest Rutherford and his colleagues in the early twentieth century, the picture of atoms consisting of tiny dense nuclei surrounded by lighter and even tinier electrons continually moving about the nucleus was well established. This picture was called the planetary model since it pictured the atom as a miniature “solar system” with the electrons orbiting the nucleus like planets orbiting the sun. The simplest atom is hydrogen, consisting of a single proton as the nucleus about which a single electron moves. The electrostatic force attracting the electron to the proton depends only on the distance between the two particles. This classical mechanics description of the atom is incomplete, however, since an electron moving in an elliptical orbit would be accelerating (by changing direction) and, according to classical electromagnetism, it should continuously emit electromagnetic radiation. This loss in orbital energy should result in the electron’s orbit getting continually smaller until it spirals into the nucleus, implying that atoms are inherently unstable.
In 1913, Niels Bohr attempted to resolve the atomic paradox by ignoring classical electromagnetism’s prediction that the orbiting electron in hydrogen would continuously emit light. Instead, he incorporated classical mechanics’ description of the atom - Planck’s ideas of quantization and Einstein’s finding that light consists of photons whose energy is proportional to their frequency. Bohr assumed that the electron orbiting the nucleus would not normally emit any radiation (the stationary state hypothesis), but it would emit or absorb a photon if it moved to a different orbit. The energy absorbed or emitted would reflect differences in the orbital energies according to this equation:
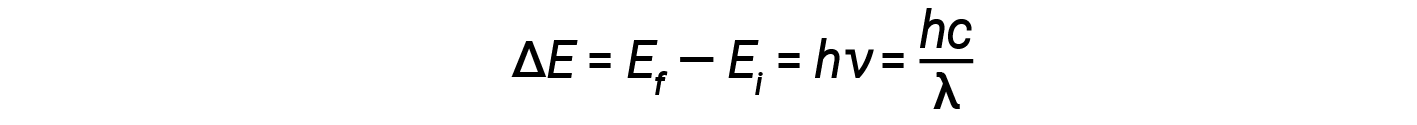
Here, h is Planck’s constant and Ei and Ef are the initial and final orbital energies, respectively. The absolute value of the energy difference is used since frequencies and wavelengths are always positive. Instead of allowing for continuous values of energy, Bohr assumed the energies of these electron orbitals were quantized.

In this expression, k is a constant comprising fundamental constants such as the electron mass and charge and Planck’s constant. Inserting the expression for the orbit energies into the equation for ΔE gives
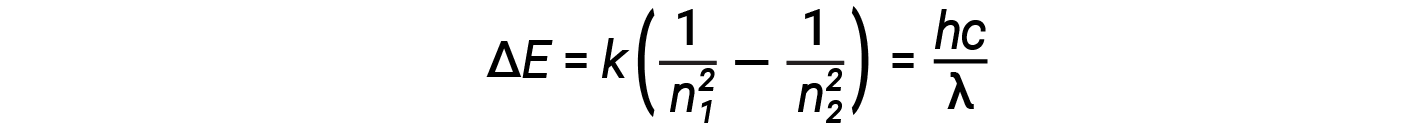
One of the fundamental laws of physics is that matter is most stable with the lowest possible energy. Thus, the electron in a hydrogen atom usually moves in the n = 1 orbit, the orbit in which it has the lowest energy. When the electron is in this lowest energy orbit, the atom is said to be in its ground electronic state (or simply ground state). If the atom receives energy from an outside source, it is possible for the electron to move to an orbit with a higher n value and the atom is now in an excited electronic state (or simply an excited state) with higher energy. When an electron transition occurs from an excited state (higher energy orbit) to a less excited state, or ground state, the difference in energy is emitted as a photon. Similarly, if a photon is absorbed by an atom, the energy of the photon moves an electron from a lower energy orbit up to a more excited one. We can relate the energy of electrons in atoms to what we learned previously about energy. The law of conservation of energy says that we can neither create nor destroy energy. Thus, if a certain amount of external energy is required to excite an electron from one energy level to another, that same amount of energy will be liberated when the electron returns to its initial state.
Since Bohr’s model involved only a single electron, it could also be applied to the single-electron ions He+, Li2+, Be3+, and so forth, which differ from hydrogen only in their nuclear charges, and so one-electron atoms and ions are collectively referred to as hydrogen-like atoms.
This text is adapted from Openstax, Chemistry 2e, Section 6.2: The Bohr Model.
From Chapter 7:

Now Playing
7.5 : The Bohr Model
Electronic Structure of Atoms
49.5K Views

7.1 : The Wave Nature of Light
Electronic Structure of Atoms
48.0K Views

7.2 : The Electromagnetic Spectrum
Electronic Structure of Atoms
52.2K Views

7.3 : Interference and Diffraction
Electronic Structure of Atoms
30.2K Views

7.4 : Photoelectric Effect
Electronic Structure of Atoms
29.1K Views

7.6 : Emission Spectra
Electronic Structure of Atoms
49.1K Views

7.7 : The de Broglie Wavelength
Electronic Structure of Atoms
25.1K Views

7.8 : The Uncertainty Principle
Electronic Structure of Atoms
22.8K Views

7.9 : The Quantum-Mechanical Model of an Atom
Electronic Structure of Atoms
41.5K Views

7.10 : Quantum Numbers
Electronic Structure of Atoms
34.0K Views

7.11 : Atomic Orbitals
Electronic Structure of Atoms
32.8K Views

7.12 : The Pauli Exclusion Principle
Electronic Structure of Atoms
33.4K Views

7.13 : The Energies of Atomic Orbitals
Electronic Structure of Atoms
23.6K Views

7.14 : The Aufbau Principle and Hund's Rule
Electronic Structure of Atoms
42.6K Views

7.15 : Electron Configuration of Multielectron Atoms
Electronic Structure of Atoms
37.4K Views
Copyright © 2025 MyJoVE Corporation. All rights reserved