An electric field suffers a discontinuity at a surface charge. Similarly, a magnetic field is discontinuous at a surface current. The perpendicular component of a magnetic field is continuous across the interface of two magnetic mediums. In contrast, its parallel component, perpendicular to the current, is discontinuous by the amount equal to the product of the vacuum permeability and the surface current. Like the scalar potential in electrostatics, the vector potential is also continuous across any boundary; hence the derivative of the vector potential is discontinuous across the boundary.
Consider two linear magnetic media with permeability μ1 and μ2, separated by an interface. The magnetic field intensity in medium 1 at a point P1 has a magnitude H1, and makes an angle α1 with the normal. Similarly, the magnetic field intensity in medium 2 at a point P2 has a magnitude H2, and makes an angle α2 with the normal.
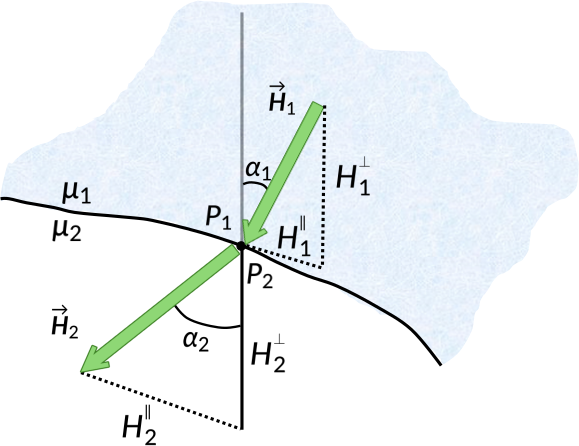
The zero divergence of the magnetic field ensures that the normal component of the magnetic field is continuous across the boundary. The magnetic field is proportional to the magnetic field intensity for linear magnetic media. Thus, the boundary conditions for the normal component of the magnetic field intensity are obtained.
Rewriting the normal components of the magnetic field in terms of the angles α1 and α2,
Since both media possess finite conductivities, free surface currents do not exist at the interface. Thus, the tangential component of the magnetic field intensity is continuous across the boundary. Again, the tangential components can be rewritten in terms of the angles α1 and α2:
Taking the ratio of normal and tangential components, a new expression is derived:
This expression is similar to the expression obtained for boundary conditions for two dielectric interfaces, except that the permittivity replaces the permeability.
If the first medium is a non-magnetic medium, like air, and the second one is a magnetic medium, the permeability of the second medium is greater than the permeability of the first medium. Thus, the angle α2 approaches ninety degrees. This implies that, for any arbitrary angle α1 that is not close to zero, the magnetic field in a ferromagnetic medium runs parallel to the interface. If the first medium is ferromagnetic and the second is non-magnetic, α2 approaches zero. This implies that if a magnetic field originates in a ferromagnetic medium, the magnetic flux lines emerge into the air medium in a direction almost normal to the interface.
Tags
From Chapter 29:

Now Playing
29.16 : Magnetostatic Boundary Conditions
Sources of Magnetic Fields
829 Views

29.1 : Magnetic Field due to Moving Charges
Sources of Magnetic Fields
8.2K Views

29.2 : Biot-Savart Law
Sources of Magnetic Fields
5.6K Views

29.3 : Biot-Savart Law: Problem-Solving
Sources of Magnetic Fields
2.4K Views

29.4 : Magnetic Field Due To A Thin Straight Wire
Sources of Magnetic Fields
4.7K Views

29.5 : Magnetic Field Due to Two Straight Wires
Sources of Magnetic Fields
2.3K Views

29.6 : Magnetic Force Between Two Parallel Currents
Sources of Magnetic Fields
3.4K Views

29.7 : Magnetic Field Of A Current Loop
Sources of Magnetic Fields
4.3K Views

29.8 : Divergence and Curl of Magnetic Field
Sources of Magnetic Fields
2.7K Views

29.9 : Ampere's Law
Sources of Magnetic Fields
3.6K Views

29.10 : Ampere's Law: Problem-Solving
Sources of Magnetic Fields
3.5K Views

29.11 : Solenoids
Sources of Magnetic Fields
2.4K Views

29.12 : Magnetic Field of a Solenoid
Sources of Magnetic Fields
3.6K Views

29.13 : Toroids
Sources of Magnetic Fields
2.8K Views

29.14 : Magnetic Vector Potential
Sources of Magnetic Fields
492 Views
See More
Copyright © 2025 MyJoVE Corporation. All rights reserved