24.15 : Second Uniqueness Theorem
Consider a region consisting of several individual conductors with a definite charge density in the region between these conductors. The second uniqueness theorem states that if the total charge on each conductor and the charge density in the in-between region are known, then the electric field can be uniquely determined.
In contrast, consider that the electric field is non-unique and apply Gauss's law in divergence form in the region between the conductors and the integral form to the surface enclosing each conductor. When integrated over the outermost boundary, the charge includes the total charge on all the conductors and the charge density in the in-between region.
If a third field is defined as the difference between the two fields, then the divergence of the third field and the integral form of the third field are zero. The product rule is used to obtain the expression for the divergence of the third field and its associated potential. The potential can be written in terms of the field, and applying that the divergence of the third field is zero gives the square of the magnitude of the electric field.

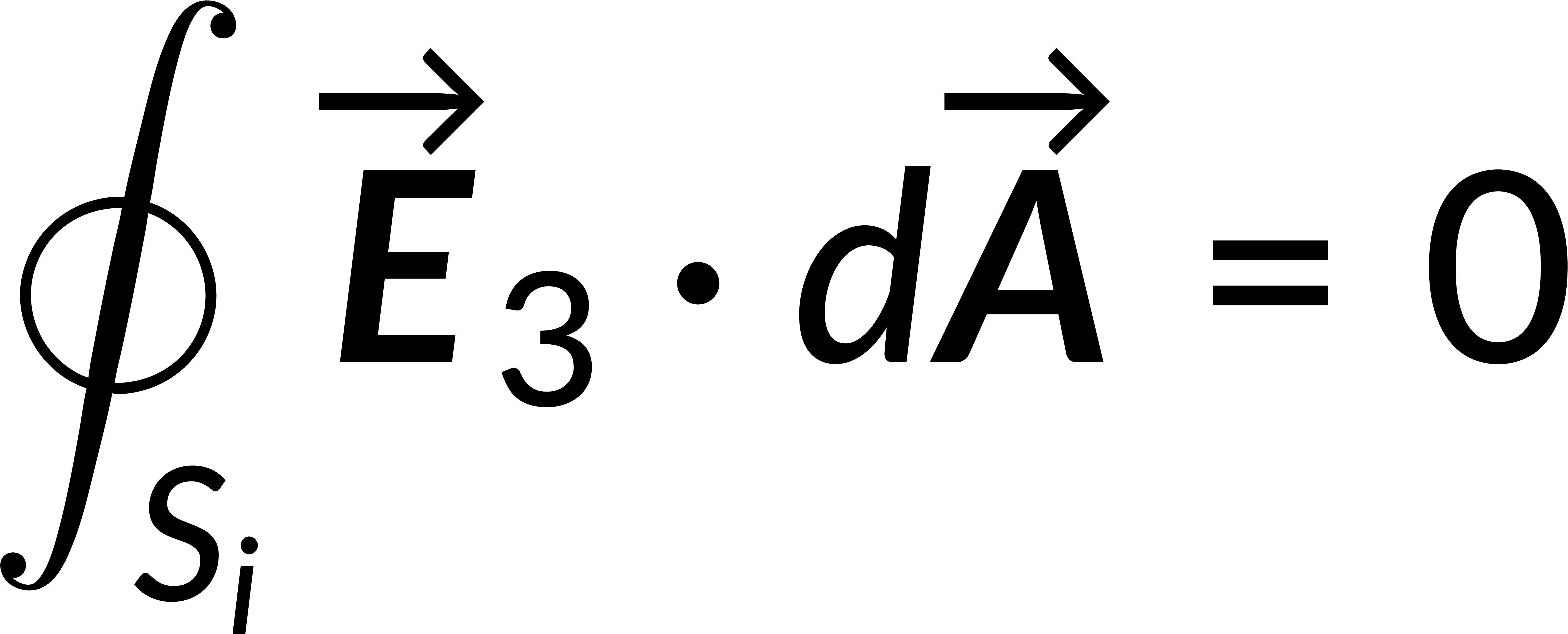

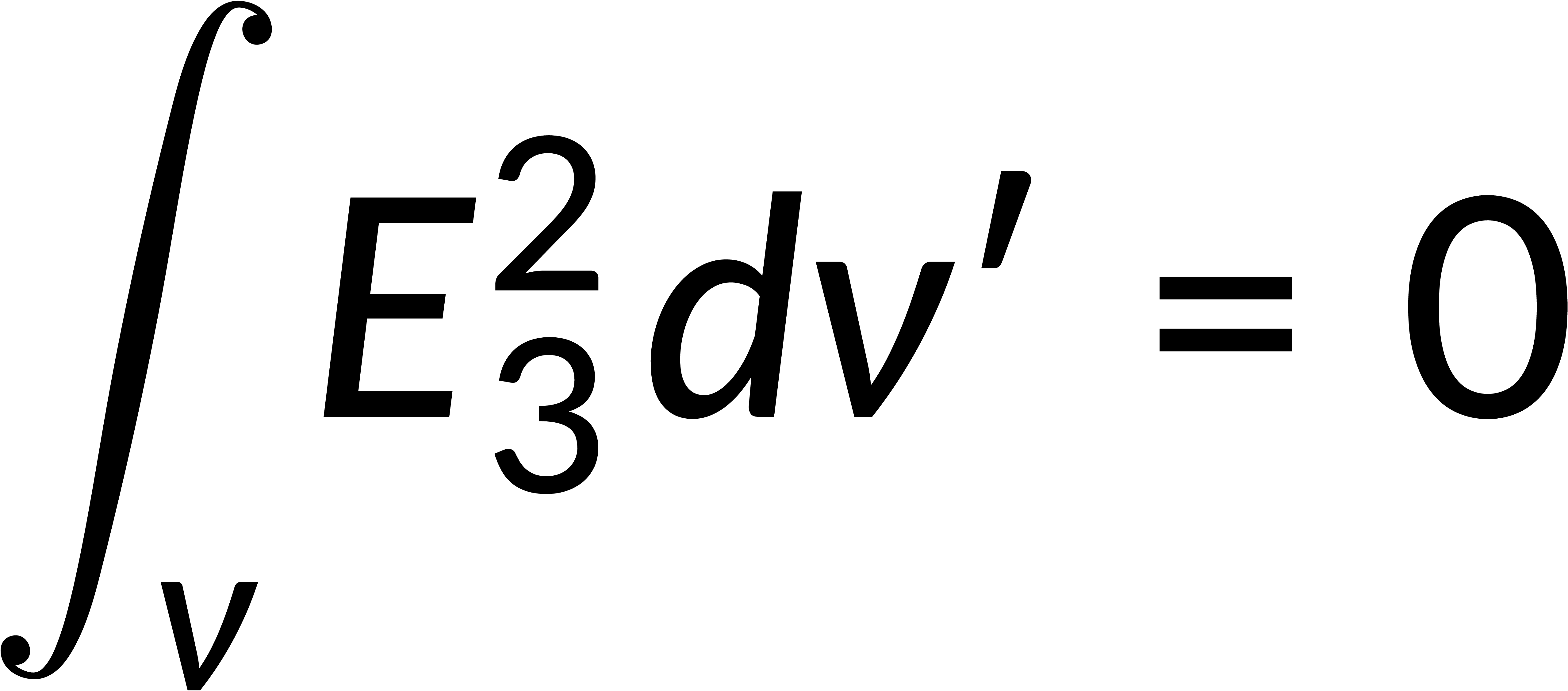
This expression is integrated over the region's volume, and the divergence theorem is applied to rewrite the volume integral as a surface integral. Recalling that the surface integral of the third field is zero implies that the magnitude of the third field is zero everywhere. This shows that the first two fields are equal, proving the solution's uniqueness.
来自章节 24:

Now Playing
24.15 : Second Uniqueness Theorem
Electric Potential
949 Views

24.1 : 电势能
Electric Potential
5.6K Views

24.2 : 均匀电场中的电势能
Electric Potential
4.5K Views

24.3 : 两点电荷的势能
Electric Potential
4.4K Views

24.4 : 电位和电位差
Electric Potential
4.2K Views

24.5 : 从电场中寻找电势
Electric Potential
4.0K Views

24.6 : 电势 I 的计算
Electric Potential
1.9K Views

24.7 : 电势计算 II
Electric Potential
1.6K Views

24.8 : 等势面和磁力线
Electric Potential
3.6K Views

24.9 : 等电位表面和导体
Electric Potential
3.3K Views

24.10 : 根据电势确定电场
Electric Potential
4.3K Views

24.11 : 泊松和拉普拉斯方程
Electric Potential
2.5K Views

24.12 : Van de Graaff 生成器
Electric Potential
1.6K Views

24.13 : 与电荷分布相关的能量
Electric Potential
1.5K Views

24.14 : 静电边界条件
Electric Potential
394 Views
版权所属 © 2025 MyJoVE 公司版权所有,本公司不涉及任何医疗业务和医疗服务。