Measurement of X-ray Beam Coherence along Multiple Directions Using 2-D Checkerboard Phase Grating

Summary

The measurement protocol and data analysis procedure are given for obtaining transverse coherence of a synchrotron radiation X-ray source along four directions simultaneously using a single 2-D checkerboard phase grating. This simple technique can be applied for complete transverse coherence characterization of X-ray sources and X-ray optics.

Abstract

A procedure for a technique to measure the transverse coherence of synchrotron radiation X-ray sources using a single phase grating interferometer is reported. The measurements were demonstrated at the 1-BM bending magnet beamline of the Advanced Photon Source (APS) at Argonne National Laboratory (ANL). By using a 2-D checkerboard π/2 phase-shift grating, transverse coherence lengths were obtained along the vertical and horizontal directions as well as along the 45° and 135° directions to the horizontal direction. Following the technical details specified in this paper, interferograms were measured at different positions downstream of the phase grating along the beam propagation direction. Visibility values of each interferogram were extracted from analyzing harmonic peaks in its Fourier Transformed image. Consequently, the coherence length along each direction can be extracted from the evolution of visibility as a function of the grating-to-detector distance. The simultaneous measurement of coherence lengths in four directions helped identify the elliptical shape of the coherence area of the Gaussian-shaped X-ray source. The reported technique for multiple-direction coherence characterization is important for selecting the appropriate sample size and orientation as well as for correcting the partial coherence effects in coherence scattering experiments. This technique can also be applied for assessing coherence preserving capabilities of X-ray optics.

Introduction

The third-generation hard X-ray synchrotron radiation sources, such as the APS at ANL, Lemont, IL, USA (http://www.aps.anl.gov), have had tremendous impacts on the development of X-ray sciences. A synchrotron radiation source generates a spectrum of electromagnetic radiations, from infrared to X-ray wavelengths, when charged particles, such as electrons, are made to move near the speed of light in a circular orbit. These sources have very unique properties such as high brightness, pulsed and pico-second timing structure, and large spatial and temporal coherence. X-ray beam spatial coherence is an important parameter of the third and fourth generation synchrotron sources and the number of experiments making use of this property has dramatically increased over the past two decades¹. The future upgrades of these sources, such as the planned Multi-bend achromat (MBA) lattice for the APS storage ring, will dramatically increase the beam coherent flux (http://www.aps.anl.gov/Upgrade/). The X-ray beam can be tuned using a crystal monochromator to achieve higher temporal coherence. The transverse coherence of synchrotron sources is significantly higher than that of laboratory based X-ray sources because of the low electron-beam emittance and long propagation distance from the source to the experimental station.

Normally, Young's double-pinhole or double-slit experiment is used to measure the spatial coherence of the beam through the inspection of the visibility of the interference fringes². To obtain the complete Complex Coherence Function (CCF), systematic measurements are needed with the two slits placed at different positions with various separations, which is, especially for hard X-rays, cumbersome and impractical. Uniformly Redundant Array (URA) can also be used for beam coherence measurement by employing it as a phase shifting mask³. Although the technique can provide the full CCF, it is not model-free. More recently, interferometric techniques based on Talbot effect were developed using the self-imaging property of periodic objects. These interferometers make use of the interferogram visibility measured at a few self-imaging distances downstream of the grating for obtaining the beam transverse coherence^4-9. Measurements of transverse coherence using two grating system is also reported⁷.

Mapping the transverse beam coherence, simultaneously along vertical and horizontal directions was first reported by J. P. Guigay et al.⁵. Recently, scientists in the Optics Group, X-ray Science Division (XSD), of APS have reported two new techniques to measure beam transverses coherence along more than two directions simultaneously using two methods: one with a checkerboard phase grating⁸, and the other with a circular phase grating⁹.

In this paper the measurement and data analysis procedures are described for obtaining the transverse coherence of the beam along the 0°, 45°, 90°, and 135° directions relative to the horizontal direction, simultaneously. The measurements were carried out at the 1-BM beamline of APS with a checkerboard π/2 phase grating. The details of this technique listed in the protocol sections include: 1) planning of the experiment; 2) preparation of the 2-d checkerboard phase grating; 3) experiment setup and alignment at the synchrotron facility; 4) performing coherence measurements; 5) data analysis. In addition, the representative results are shown to illustrate the technique. These procedures can be carried out at many synchrotron beamlines with minimum changes on the grating design.

Protocol

1. Planning of the Experiment

1. Identify the synchrotron beamline. Contact beamline scientist to find the appropriateness of the experiment at that beamline.
   NOTE: Experiments reported in this manuscript were performed at the 1-BM-B beamline, which is dedicated to optics and detectors testing, under XSD of APS.
2. Submit a user proposal and beam time request.
3. Work out the details of the experiment with the beamline scientist and specify the required instruments including motorized stages for the grating and detector alignment, 2-dimensional detector (CCD or CMOS), long translation stage covering the least and farthest distances needed between the detector and the phase grating.
4. Prepare for the beam time by following the instructions provided in the relevant website. Complete the safety trainings and necessary experimental safety assessment form.

2. Preparation of the 2-D Checkerboard Phase Grating

1. Determine the period of the grating, p, which is related to the period of the interferogram pattern, p_θ, along different transverse direction angle θ. The visibility values, V_θ(d), of the interferogram along different θ angle oscillate as a function of the grating-to-detector distance, d.
   For a 2-D checkerboard π/2 phase grating, V_θ(d) peaks at distances,
   
   with n = 1, 2, 3… and λ the photon wavelength. The interferogram pattern has a characteristic period of p_θ = p/√2 along the diagonal direction of the square blocks and a period of p_θ = p/2 along the edge of the square blocks. The choice of p thus relies on the following criteria.
2. Make sure at least several V_θ(d) peaks are within the largest grating-to-detector distance, or the space limit of the experimental station, d_max. To satisfy d_n,θ < d_max, it follows
   
   For n = 5, d_max = 1 m, λ = 0.06888 nm (18 keV), it gives p_θ < 3.9 µm.
3. Within d_max, make sure that the height of the V_θ(d) peak at the largest distance d_n,θ is less than a factor γ of that of the first V_θ(d) peak at d_1,θ in order to have an accurate Gaussian decay function fitting. Hence, γ = V_θ,n(d) / V_θ,1(d) which is the ratio of the n^th peak visibility to the first peak. For an X-ray source following Gaussian intensity distribution with the coherence length, ξ_θ, the period of a π/2 phase grating needs to satisfy
   
   for instance, with γ = 10%, ξ_θ = 5 µm and parameters above, it gives p_θ > 2.4 µm.
4. Ensure that the period of the interferogram pattern, p_θ, is a few times larger than the spatial resolution of the detector by choosing the correct detector systems.
5. Determine the thickness, T, of the grating required for a phase shift of, φ, at the X-ray photon wavelength, λ, using
   
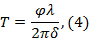
   where δ is the refractive index decrement of the phase shifting material. For example, the refractive index decrement for Au is 9.7×10^-6 for 18 keV. The Au thickness for φ = π/2 phase grating is thus 1.8 µm.
6. Fabricate the phase grating by electroplating Au into a patterned polymer mold on a silicon nitride (Si₃N₄) window.
   NOTE: The procedure for preparation of silicon nitride (Si₃N₄) window substrate and fabrication of the grating structure are presented below.
   1. Prepare the substrate by first releasing the Si₃N₄ membrane to form the X-ray transparent window.
   2. Acquire silicon (Si) wafers with low stress (<250 MPa) Si₃N₄ deposited on both sides of the wafer from a vendor.
   3. Load the wafer into a magnetron sputtering deposition system to deposit Cr and Au to act as an electroplating base.
   4. Deposit 5 nm of Cr then 30 nm of Au on one side of the wafer, following manufacturer's directions.
      NOTE: The deposition processes from the system manufacturer will include information such as deposition rate.
   5. Unload wafer from deposition tool. Use the side of the wafer deposited with Cr and Au for grating fabrication.
   6. Determine the total size of the grating and then design a photolithography mask to pattern membranes slightly larger. Use the design to acquire a photolithography mask by purchasing from a vendor or fabricate the photolithography mask.
   7. Spin a 3-µm thick layer of photoresist on the back side of the wafer where there is no Cr and Au coating. Expose the resist with a UV lithography tool for 20 sec using the designed photolithography mask. Develop the exposed resist in aqueous alkaline developer solution for 30 sec then rinse with deionized water and dry with flowing N₂.
   8. Load the wafer into a reactive ion etching (RIE) tool with patterned photoresist facing the chamber. Use CF₄ plasma to etch the exposed Si₃N₄ following tool instructions.
   9. Evacuate the etching chamber and input etch recipe into RIE tool. Run the recipe until the Si₃N₄ layer is etched completely and the Si layer is exposed in the pattern.
   10. Etch the exposed Si on the wafer backside by submerging into 30% KOH solution heated to 80 °C for about 8 hr. Etch rate is approximately 75 µm/hr using the stated recipe.
   11. After Si etch is finished, rinse with deionized water and dry with flowing N₂. The sample is ready for grating fabrication.
7. Fabricate the electroplating mold for the phase grating using the following steps.
   1. Design the square checkerboard grating pattern and compensate for pattern biasing by reducing the exposed square pattern size by 100-250 nm. Include a >50-µm wide frame around the grating pattern for thickness confirmation later in the process.
   2. Load the sample into a resist spin coater and deposit poly (methyl methacrylate) (PMMA) positive resist solution on the grating side of the sample. Run the resist spin coater to form a 2 to 3.5-µm thick resist film depending on desired final grating thickness.
      NOTE: Spin curves with information on spin speed versus film thickness are provided by the PMMA solution vendor or can be determined empirically.
   3. Load the wafer into a 100 keV electron beam lithography system.
   4. Calibrate tool for exposure with a large exposure current greater than 10 nA.
   5. Expose the PMMA resist using a 100 keV e-beam lithography tool to create the grating pattern, where areas exposed will be removed in the development step. Use an exposure dose range of 1,100-1,250 µC/cm² depending on resist thickness.
   6. Unload the sample from the tool.
   7. Develop the exposed resist by submerging in a 7:3 (by volume) isopropyl alcohol (IPA): deionized water solution for 30-40 sec with gentle swirling. Rinse with IPA, and then dry with flowing N₂. Ensure the PMMA was fully developed by looking at exposed area with an optical microscope.
   8. Load the sample into a RIE tool with PMMA pattern facing the chamber.
   9. Evacuate the etching chamber and input descum etch recipe into RIE tool. The descum process is a short (<30 sec) O₂ plasma based etch to remove any residual PMMA from the exposed grating area.
8. Finish the Au grating by electroplating into the fabricated mold using the following steps.
   1. Make sure the electroplating mold thickness by scanning the probe of a profilometer across the frame included for thickness confirmation.
   2. Submerge the sample into the Au-sulfite electroplating solution heated to 40 °C. The electroplating setup is composed of a beaker filled with the electroplating solution, a constant current DC power supply, and a Pt mesh anode.
   3. Determine the plating area of the sample by calculating the exposed Au in the exposed pattern, then calculate current for the desired current density, which is the primary variable used to set the deposition rate.
   4. Calculate plating time to reach desired grating thickness using the plating rate determined by the applied current density.
   5. Turn on the DC power supply to apply the determined current on the sample, acting as a cathode, and plate for approximately half the total plating time.
   6. Measure the plating thickness using the same method used in step 2.8.1.
   7. Turn on the DC power supply to electroplate Au into the PMMA mold and electroplate to the desired grating thickness, taking into account the plated height measured in step 2.8.6.
9. Remove the polymer mold using a heated solvent by submerging the sample. Then inspect with an optical microscope and a scanning electron microscope (SEM) to confirm grating period, duty cycle, and grating thickness.
   NOTE: Have two 2-D checkerboard phase gratings (one for the experiment and one as a spare) ready, a few days before the experiment begins.

3. Experiment Setup and Alignment at the Synchrotron Facility

1. Request the beamline scientist to set the X-ray beam energy or wavelength to the desired value that matches the phase grating. Routinely used X-ray energies at the APS 1-BM beamline are between 6 and 28 keV. In this case, tune the photon energy to 18 keV.
2. Select the desired objective lens for the detector system. Here, use a Coolsnap HQ2 CCD detector with 1,392 × 1,040 imaging pixels of 6.45 × 6.45 µm² pixel size. To resolve the smallest interference pattern, use an EC plan Neofluar 10× objective. The effective pixel size of the detector system including the magnification effect of microscopic objective is thus 0.64 µm. The estimated spatial resolution is about 2 µm, which is mainly due to the point spread function of the detector system.
3. To set the rough focusing of the detector system, place the scintillator (lutetium-yttrium oxyorthosilicate, 150-µm thick) at the 'working distance' from the lens (~5.2 mm for the used system). At first, set the focus under ambient light by monitoring the images acquired under 'continuous mode' as the scintillator position is adjusted using a pico-motor.
4. Move the 2-D detector into the X-ray beam, by using vertical and horizontal stages align the center of the detector to the beam center.
5. Place a 'phase sample', for example a piece of Styrofoam, into the X-ray beam. Perform the fine focusing of the detector system by observing the scattering pattern from the phase sample and adjusting the scintillator position until the highest image sharpness.

4. Performing Coherence Measurements

1. Place the 2-D checkerboard grating into the X-ray beam where the coherence of the beam is to be measured. In this case it is at 34 meters from the bending magnet source.
2. Adjust the plane of the 2-D checkerboard phase grating to be perpendicular to the direction of the X-ray beam propagation.
3. Center the grating to the X-ray beam by using the motorized stages and looking at the images acquired under detector continuous mode.
4. Rotate the grating around the X-ray beam propagation direction (y) so that the diagonal direction of the checkerboard pattern is along the desired transverse beam direction. In this case, align the diagonal directions of the checkerboard (preferred measuring directions) in the horizontal and vertical directions of the beam. Fine tune the grating rotations around the other two axes (x and z) to ensure its perpendicularity to the X-ray beam, which is achieved by maximizing the interferogram periods in both the horizontal and vertical directions.
5. Move the detector system as close as physically possible to the phase grating along the beam propagation direction. In this study, use a distance of 43 mm.
6. Calculate the smallest period in the interference pattern. The π/2 checkerboard grating with period p = 4.8 µm will generate an interference pattern with p_θ = 3.4 µm and p_θ = 2.4 µm (smallest period) along the diagonal and the non-diagonal directions of the checkerboard pattern, respectively. Estimate the number of data points needed in-between V_θ(d) peak positions given by Equation (1) to obtain a smooth curve.
7. Select the appropriate exposure time for each interferogram, four seconds in this case.
8. Record interferograms with the same exposure time (e.g., 4 sec) at different grating-to-detector distances. Choose the exposure time based on the beam intensity level. Starting from the minimum grating-to-detector distance (43 mm), move the detector downstream of the X-ray by small intervals (10 mm determined based on step 4.6) and record an interferogram at each detector position until the maximum possible grating-to-detector distance (750 mm).
9. Acquire dark-frame images with the same exposure time (4 sec) but turn off the X-ray beam and keep all other experimental conditions the same.

5. Data Analysis

NOTE: There is currently no standard software available for the data analysis.

1. Using the selected image processing program, read in the dark-frame image(s) and the data image. Correct the data image by subtracting the (averaged) dark-frame image.
2. Fourier transform the dark-frame corrected image, which produces visible harmonic peaks in the horizontal (θ = 0º), vertical (θ = 90º) as well as θ = 45° and θ = 135° directions.
3. Crop the 0^th order harmonic image centered at the 0^th order peak. The length and width of the image equal to the distances between the 0^th and 1^st order peaks along the horizontal and vertical directions, respectively. Similarly, obtain the 1^st order harmonic images of the same length and width along the transverse direction of interest.
4. Inverse Fourier Transform (IFT) the cropped harmonic images. Ratio of the mean of the amplitudes of the IFT image from the 1^st order harmonic image along any transverse direction to that of the IFT image from the 0^th order harmonic image gives the visibility along that direction.
   Note that this process is valid if few high-frequency components exist in the measured interferogram. Otherwise, one can use the corresponding harmonic peak intensities of the Fourier transform images from step 5.4 instead. Due to the beam divergence, the harmonic peak positions will change gradually at different grating-to-detector distances. Therefore, a correction to p'_θ at each distance or a peak finding process is needed.
5. Repeat step 5.1-5.4 for all the measured images at different grating-to-detector distances and save the visibility value of each image.
6. Plot the visibility V_θ(d) as a function of the grating-to-detector distance. Identify data points at V_θ(d) peaks. Note that the full curve was measured just to better identify the peak positions given by Equation (1). Manually select peak data points as well as adjacent data points on either side of each peak.
7. Draw Gaussian fitting function for the selected data points. Extract standard deviation, σ_θ, of the Gaussian fitting function.
8. Obtain the transverse coherence length, ξ_θ, using
   

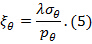
Results

While detailed experimental and simulation results could be found elsewhere⁸, this section only shows selected results to illustrate the above measurement and data analysis procedures. Figure 1 represents the experiment setup at the APS 1-BM-B beamline. The beam size is defined by a 1×1 mm² slit placed upstream of the Double Crystal Monochromator (DCM) and 25 m from the bending magnet source. The DCM is tuned to output photon energy of 18 keV. The X-ray beam passes through seve...

Discussion

Figure 5 shows the estimated transverse coherence length along all four directions. Clearly, the 90° direction has higher ξ_θ compared to 0° direction. Since the beamline optics has negligible effect on the beam coherence at the grating relative location, the measured coherence area is inversely proportional to the source size area. The presented X-ray beam coherence measurement technique maps this accurately which can be shown as an ellipse with its major axis alon...

Materials

Name	Company	Catalog Number	Comments
1-BM-B bending magnet x-ray source	Advanced photon Source/ Argonne National Lab		http://www.aps.anl.gov/Xray_Science_Division/Optics/Beamline/
LYSO Scintillator	Proteus Inc		http://www.apace-science.com/proteus/lyso.htm#top
Coolsnap HQ2 CCD detector	Photometrics		http://www.photometrics.com/products/ccdcams/coolsnap_hq2.php
ATC 2000 UHV sputtering deposition system	AJA International Inc		http://www.ajaint.com/systems_atc.htm
MICROPOSIT S1800 photoresist	Dow
MICROPOSIT 351 developer	Dow
MA/BA6 lithography system	SUSS MicroTec		http://www.suss.com/en/products-solutions/products/mask-aligner/maba6/overview.html
Spin coater WS-400-6NPPB	Laurell Technologies Corporation		http://www.laurell.com/spin-coater/?model=WS-400-6NPP-LITE
JBX-9300FS electron beam lithography system	JEOL		http://www.jeolusa.com/PRODUCTS/PhotomaskDirectWriteLithography/ElectronBeamLithography/JBX-9500FS/tabid/245/Default.aspx
CS-1701 RIE system	Nordson March		http://www.nordson.com/EN-US/DIVISIONS/MARCH/PRODUCTS/LEGACY/Pages/CS-1701-Anisotropic-RIE-Plasma-System.aspx
Techni Gold 25E	Technic		http://www.technic.com/eu/applications/industrial/industrial-chemistry/plating-chemistry
Dektak-8 surface profiler	Bruker		http://brukersupport.com/ProductDetail/1136
MICROPOSIT 1165 remover	Dow