Wicking Tests for Unidirectional Fabrics: Measurements of Capillary Parameters to Evaluate Capillary Pressure in Liquid Composite Molding Processes

Summary

An experimental method to measure geometrical parameters and the apparent advancing contact angles describing capillary wicking in unidirectional synthetic and natural fabrics is proposed. These parameters are mandatory for the determination of the capillary pressures that must be taken into account for liquid composite molding (LCM) applications.

Abstract

During impregnation of a fibrous reinforcement in liquid composite molding (LCM) processes, capillary effects have to be understood in order to identify their influence on void formation in composite parts. Wicking in a fibrous medium described by the Washburn equation was considered equivalent to a flow under the effect of capillary pressure according to the Darcy law. Experimental tests for the characterization of wicking were conducted with both carbon and flax fiber reinforcement. Quasi-unidirectional fabrics were then tested by means of a tensiometer to determine the morphological and wetting parameters along the fiber direction. The procedure was shown to be promising when the morphology of the fabric is unchanged during capillary wicking. In the case of carbon fabrics, the capillary pressure can be calculated. Flax fibers are sensitive to moisture sorption and swell in water. This phenomenon has to be taken into account to assess the wetting parameters. In order to make fibers less sensitive to water sorption, a thermal treatment was carried out on flax reinforcements. This treatment enhances fiber morphological stability and prevents swelling in water. It was shown that treated fabrics have a linear wicking trend similar to those found in carbon fabrics, allowing for the determination of capillary pressure.

Introduction

During impregnation of fibrous reinforcements in liquid composite molding (LSM) processes, the resin flow is driven by a pressure gradient. Capillary effects have an additional effect that can compete with the pressure gradient, depending on the process parameters. Their influence on the process thus has to be evaluated^1,2. This can be done by defining an apparent capillary pressure, P_cap, modifying the initial pressure gradient³. This parameter may subsequently be inserted into numerical models in order to simulate flows during processes and to accurately predict void formation⁴.

The spontaneous impregnation of a fabric by a liquid (wicking) can be described by the Washburn equation⁵. Originally, the Washburn equation described the capillary rise of a liquid in a tube. This equation was then extended for porous structures, such as fibrous reinforcements, that can be approximated to a capillary tube network. Considering a cylindrical sample holder with a radius, R, filled with a porous medium, the Washburn equation was modified in the form of squared mass gain (m²(t)) over time, as follows⁶:

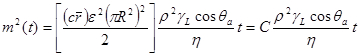        (1)

where c is a parameter that accounts for tortuosity, ṝ is the mean pore radius, and ε = 1-V_f is the porosity (V_f being the fiber volume ratio). All parameters in the square brackets concern the morphology and configuration of the porous medium, and they can be consolidated into a constant, C, referred to as the "geometric porous medium factor." The other parameters express the dependence of wicking on the interactions between the medium and the liquid (through ρ, η, and γ_L, which are, respectively, the density, viscosity, and surface tension of the liquid, and through θ_a, an apparent advancing contact angle).

In parallel, the flow through a porous medium is usually modelled with the well-known Darcy law⁷, which relates an equivalent fluid velocity, v_D, to the pressure drop through the permeability of the medium, K, and the liquid viscosity, η. This equation also allows for the expression of the mass gain over a square root of time and thus for the consideration of the equivalence between the two equations. From this equivalence between the Washburn equation and the Darcy law, the capillary pressure was then defined as follows⁸:

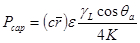        (2)

Here, the main focus is to describe the experimental procedure to measure the geometric factors and the apparent advancing contact angles for unidirectional fabrics, with the aim of determining the capillary pressure. This method relies on using a tensiometer to perform wicking tests (Figure 1). A tensiometer is a microbalance with a resolution of 10 µg that measures the liquid mass either forming a meniscus around a solid or ascending a fibrous medium. Wicking tests were carried out considering a one-dimensional characterization (direction along the fibers)^8,9. Quasi-unidirectional fabrics used to validate the procedure were carbon uni-directional (UD) fabrics at a V_f = 40%. Once the method was validated, flax fabrics were submitted to a thermal treatment that modifies the wetting behavior of fibers⁶, and wicking tests were performed with different fiber volume ratios (from 30% to 40%) for both untreated and treated flax fabrics. To determine morphological and wetting parameters, at least two wicking tests are mandatory: the first one with a totally-wetting liquid, like n-hexane, to determine C (Equation 1), and the second one with the liquid of interest, to determine the apparent advancing contact angle once C is known. In the first approach, water was used to evaluate the procedure.

This method can be applied to different fabrics and liquids, allowing for the evaluation of the influence of material geometry (morphology of fabrics), porosity (different fiber volume ratios), and viscosity and surface tension of liquid on the capillary impregnation phenomena. It is obvious that the procedure according to the Washburn theory (Equation 1) can be adopted only if wicking curves (m²(t)) recorded by the tensiometer have a linear trend. This means that the parameters in Equation 1 must remain constant during the entire wicking process. If this is not the case, as for flax reinforcements in water, because fibers undergo swelling^10,11, the Washburn equation should be modified to include the effect of swelling in order to describe the tests properly⁹. Treated fabrics were found to be less sensitive to water sorption⁹. Geometric factors and wetting parameters can be measured from linear fits, allowing for the calculation of the capillary pressure, P_cap.

Protocol

Caution: Consult all relevant material safety data sheets. Chemicals used for the tests are toxic and carcinogenic. Use personal protective equipment (safety glasses, gloves, lab coat, full-length pants, and closed-toe shoes).

1. Setup for Tests

1. Preparation of samples
   1. Cut strips of fabric along the direction perpendicular to the fibers (in order to test wicking in the fiber direction).
      NOTE: The lengths of the strips are calculated in order to obtain a defined fiber volume ratio. For carbon fabrics, to obtain V_f = 40%, the length of the strips was 150 mm. For untreated and treated flax, to achieve the same V_f, the length was 365 mm. The width of each strip will be equal to the height of sample holder, which is 20 mm (Figure 1).
   2. Roll the strips tightly to allow their insertion into the cylindrical sample holder of R = 6 mm.
   3. Add a thin paper filter between the sample holder and the sample reinforcements (in order to suppress the effect of the sample holder on the wicking). The maximum thickness of the paper filter should be 0.1 mm.
   4. Insert the sample into the cylinder and screw the drilled cap at the bottom and the piston at the top in order to ensure compaction.
   5. Clamp the sample holder with the fabric to the tensiometer.
2. Preparation of liquids
   1. Fill a vessel with the liquid test and place it into the specific receptacle of the tensiometer. Use vessels made of borosilicate glass and with a diameter of 70 mm.
   2. For the first test (step 2.1), use n-hexane. For the second test (step 2.3), use water. Ensure that the liquid in the vessel reaches a height of at least 12 mm.
3. Experimental parameters
   1. Set the surface detection threshold to 8 mg and the translation speed of the liquid vessel at 0.5 mm/s for detection of the liquid.

2. Wicking tests

NOTE: After the preparation of samples and the setup of the tensiometer parameters, the wicking tests can start. The liquid vessel moves up until the liquid is in contact with the sample holder. Then, liquid rises into the sample holder, and the tensiometer measures the squared liquid mass gain over time. Data are recorded by the software provided with the tensiometer. One curve of mass against time is then visualized for each wicking test.

1. Initial test for determining the geometric factor:
   1. Use a totally-wetting liquid (for which the contact angle is 0°), such as n-hexane.
   2. Stop the wicking test when the visualized curve achieves a constant value. This indicates that the liquid has reached the top of the sample holder and thus that the wicking is complete.
   3. Fit the linear trend of the wicking curve (m²(t)) with the Washburn equation:
              (3)
      Since the advancing contact angle is supposed 0° with n-hexane, from the slope of linear fit, determine the geometric constant, C (mm⁵).
      NOTE: All tests were carried out under standard conditions at 20 °C. A change in temperature will modify the liquid surface tension and the results.
2. Cleaning of sample holder for the following tests
   NOTE: After removing the wet fabric, the sample holder has to be cleaned perfectly to prevent errors in the following measurements.
   1. Immerse the sample holder in a vessel with sulfochromic acid (50% of a saturated solution of potassium dichromate and 50% of concentrated sulfuric acid) for 30 sec.
   2. Rinse it with distilled water and then dry it.
3. Second test for determining the apparent advancing contact angle
   1. Use the liquid for which the advancing contact angle has to be measured with a new, identical, and dry fabric sample.
      NOTE: Water was used in order to validate the method.
   2. Stop the wicking test when the visualized curve achieves a constant value. This indicates that the liquid has reached the top of the sample holder and that the capillary rise is complete.
   3. Fit the linear part of the wicking curve (m²(t)) with the Washburn equation (Equation 3), since the constant, C, is already known due to the first test (step 2.1), with the slope of linear fit determining the advancing contact angle, θ_a (°).
      NOTE: All tests were carried out under standard conditions at 20 °C. A change in temperature will modify the liquid surface tension and the results.
4. Evaluation of the liquid weight contribution due to the sample holder
   NOTE: The tensiometer, as a microbalance, measures the total mass of liquid, including both the liquid ascending in the fabric and the contribution of the external meniscus on the sample holder and the wicking in the filter. Those contributions must be isolated.
   1. Put the same amount of filter paper as used in step 1.1.3 into the sample holder and repeat steps 2.1.1-2.1.2.
   2. Subtract the constant value obtained (m²) from the data recorded in step 2.1.3 and shift the curve to assess the correct evaluation of the geometric constant, C.
   3. Fill the sample holder with only the filter paper and repeat steps 2.3.1-2.3.2.
   4. Subtract the constant value obtained (m²) from the data recorded in step 2.3.3 and shift the curve to assess the correct evaluation of the advancing contact angle, θ_a.

Results

Curves of mass gain during wicking obtained with the tensiometer for carbon and untreated and treated flax fabrics are shown in Figures 2 and 3. All curves are shown after the subtraction of both weights of the external meniscus due to the sample holder and filter paper and are shifted to zero.

It is possible to observe from the plots in Figure 2 that, with both n-hexane and wat...

Discussion

The critical steps in the protocol relate to the preparation of the samples. Firstly, the rolled sampled have to be tight in order to make the assumption of a homogeneous fiber volume ratio. If there is a tightness gradient in the sample, the Washburn equation^5,6 cannot be used to fit the wicking curves. In addition, the boundary conditions between the fabric and the sample holder are difficult to control. Thus, the filter paper (1.1.3) must also be carefully ins...

Materials

Name	Company	Catalog Number	Comments
Carbon UD fabrics	Hexcel	48580
Flax UD fabrics	Libeco	FLAXDRY UD 180
n-Hexane	Sigma Aldrich
Sulfochromic acid	home made		toxic and corrosive
Filter paper	Dataphysic	FP11
Tensiometer	Dataphysic	DCAT11