If the rotational definitions are compared with the definitions of linear kinematic variables from motion along a straight line and motion in two and three dimensions, we can observe a mapping of the linear variables to the rotational ones.
When comparing the linear and rotational variables individually, the linear variable of position has physical units of meters, whereas the angular position variable has dimensionless units of radians, as it is the ratio of two lengths. The linear velocity has units of m/s, and its counterpart, the angular velocity, has units of rad/s.
In the case of circular motion, the linear tangential speed of a particle at a radius r from the axis of rotation is related to the angular velocity by the relation
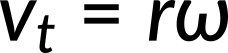
This could also apply to points on a rigid body rotating about a fixed axis. Here, only circular motion is considered. In a circular motion, both uniform and nonuniform, there exists a centripetal acceleration. The centripetal acceleration vector points inward from the particle executing circular motion toward the axis of rotation.
Thus, in a uniform circular motion, when the angular velocity is constant and the angular acceleration is zero, we observe a linear acceleration, that is, centripetal acceleration, since the tangential speed is a constant.
This text is adapted from Openstax, University Physics Volume 1, Section 10.3: Relating Angular and Translational Quantities.
Du chapitre 10:

Now Playing
10.5 : Relating Angular And Linear Quantities - I
Rotation et systèmes matériels
6.4K Vues

10.1 : Vitesse angulaire et déplacement
Rotation et systèmes matériels
13.9K Vues

10.2 : Vitesse angulaire et accélération
Rotation et systèmes matériels
8.7K Vues

10.3 : Mouvement de rotation uniformément varié - I
Rotation et systèmes matériels
6.5K Vues

10.4 : Mouvement de rotation uniformément varié - II
Rotation et systèmes matériels
5.8K Vues

10.6 : Établir une relation entre le mouvement linéaire et le mouvement angulaire - II
Rotation et systèmes matériels
5.3K Vues

10.7 : Moment d'inertie
Rotation et systèmes matériels
11.1K Vues

10.8 : Moment d'inertie et énergie cinétique de rotation
Rotation et systèmes matériels
7.1K Vues

10.9 : Moment d'inertie : calculs
Rotation et systèmes matériels
6.6K Vues

10.10 : Moment d'inertie d'un solide composé
Rotation et systèmes matériels
6.0K Vues

10.11 : Théorème des axes parallèles (théorème de Huygens)
Rotation et systèmes matériels
6.3K Vues

10.12 : Théorème de l’axe perpendiculaire
Rotation et systèmes matériels
2.6K Vues

10.13 : Transformation vectorielle dans les systèmes de coordonnées en rotation
Rotation et systèmes matériels
1.3K Vues

10.14 : Coriolis Force
Rotation et systèmes matériels
2.9K Vues