항온 풍속 측정: 난류 경계층 흐름 연구 도구
Overview
출처: 샤오펑 리우, 호세 로베르토 모레토, 제이미 도라도 항공우주공학과, 샌디에이고 주립대학교, 샌디에이고, 캘리포니아
경계층은 유동장에 침지된 고체 바디의 표면에 바로 인접한 얇은 유동 영역입니다. 이 지역에서점성 전단 응력과 같은 점성 효과는 유체와 고체 표면 사이의 마찰의 영향으로 인해 유동이 지연됩니다. 경계 층 의 외부, 흐름은 비스타, 즉, 마찰, 열 전도 또는 질량 확산으로 인한 소멸 효과가 없다.
경계 층 개념은 1904 년 Ludwig Prandtl에 의해 도입되었으며, 이는 견고한 몸위에 흐르는 처리를위한 Navier-Stokes (NS) 방정식에 상당한 단순화를 가능하게합니다. 경계 층 내부의 NS 방정식은 경계 레이어 방정식으로 감소되고 경계 층 외부에서는 NS 방정식의 단순화된 버전인 오일러 방정식에 의해 흐름을 설명할 수 있다.

그림 1. 플랫 플레이트를 통해 경계 층 이 개발됩니다.
경계 층 개발을위한 가장 간단한 경우는 발생률이 0 각도로 플랫 플레이트에서 발생합니다. 플랫 플레이트의 경계 층 개발을 고려할 때 경계 층 외부의 속도는 일정하므로 벽을 따라 압력 그라데이션이 0으로 간주됩니다.
고체 체면에서 자연적으로 발생하는 경계 층은 일반적으로 다음 단계를 거칩니다: 첫째, 라미나르 경계 층 상태; 둘째, 전환 상태 및 세 번째, 난류 경계 층 상태. 각 주에는 경계 레이어의 흐름 구조를 설명하는 자체 법률이 있습니다.
경계 층의 개발 및 구조에 대한 연구는 이론적 연구와 실용적인 응용 분야에서 매우 중요합니다. 예를 들어, 경계 층 이론은 선박, 항공기 및 터보 머신의 블레이드에 피부 마찰 드래그를 계산하기위한 기초입니다. 피부 마찰 드래그는 경계 층 내의 신체 표면에 생성되며 직접 접촉하는 유체 입자를 통해 표면에 가해지는 점성 전단 응력 때문입니다. 피부 마찰은 표면의 유체 점도 및 국소 속도 그라데이션에 비례하여 표면 정상 방향으로 기울어집니다. 피부 마찰 드래그는 전체 표면에 존재하므로 비행기 날개와 같은 넓은 영역에 걸쳐 유의하게됩니다. 또한 난류 유체 흐름은 더 많은 피부 마찰 드래그를 생성합니다. 거시적 난류 유체 모션은 높은 운동량으로 유체 입자를 표면으로 끌어내려 경계 층 내의 운동 전달을 향상시킵니다.
이 데모는 혼합 또는 데딩과 같이 흐름이 불규칙하고 평균 흐름에 변동이 중첩되는 플랫 플레이트 위에 난류 경계 층에 중점을 둡니다. 따라서, 난류 경계 층의 어느 시점에서의 속도는 시간의 함수이다. 이 데모에서는 일정한 온도 핫 와이어 적임제 또는 CTA가 경계 층 조사를 수행하는 데 사용됩니다. 이어서, 절제차트 방법은 난류 경계층에서 피부 마찰 계수를 계산하는 데 사용될 것이다.
Procedure
1. 핫와이어 시스템 동적 응답 결정
이 절차의 목적은 방혈계 시스템이 유동 신호 변화에 얼마나 빨리 대응할 수 있는지 이해하는 것입니다. 이 기능은 사각 파를 적용하여 신호가 켜지고 끌 때 주파수 응답을 측정하여 측정합니다.
- 지지축을 사용하여 윈드터널 내부CTA 시스템의 핫 와이어 프로브를 보호합니다.
- DC 전원 공급 장치, 신호 발생기 및 진동기를 설정하고 그림 2(a)에 표시된 대로 연결합니다. 신호 발생기는 휘트스톤 브리지에 사각형 파동 입력을 공급하며 출력 파형은 오실로스코프에 시각화됩니다.
- 핫와이어 전원 공급 장치, 오실로스코프 및 신호 발생기를 켭니다.
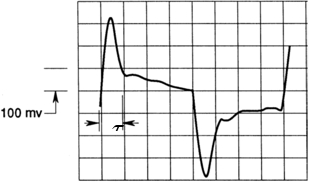
- 150mV 진폭과 10kHz 주파수로 사각 파를 출력하기 위해 신호 발생기를 설정합니다.
- 오실로스코프의 출력 신호를 관찰하여 출력 파형태 주파수 및 진폭이 올바른지 확인합니다.
- 테스
Results
CTA는 다른 공기 속도로 핫 와이어의 전압을 측정하여 프로토콜의 섹션 2에서 보정되었습니다. 이 데이터는 측정된 변수, 전압 및 간접 변수인 공기 속도 사이의 수학적 관계를 결정하는 데 사용되었습니다. 실험 데이터를 속도에 맞게 수학적 관계에 맞추는 방법에는 여러 가지가 있으며, 그 중 일부는 부록에서 다룹니다. 수학적 관계가 결정되면 CTA를 추가 실험에서 전압?...
Application and Summary
데모는 표면 위로 난류 흐름을 연구하는 데 사용되는 강력한 도구인 일정한 온도 무모법을 사용하는 방법을 보여 주며, 이 특정 경우 플랫 플레이트였습니다. 이 방법은 PIV, PTV 및 LDV와 같은 다른 방법보다 간단하고 저렴하며 높은 시간적 해상도를 제공합니다. 난류 경계 층에 핫 와이어 무모트리를 적용하면 난류 흐름의 동작을 입증하는 비용 효과적이고 실습 접근 방식을 제공합니다.
Log in or to access full content. Learn more about your institution’s access to JoVE content here
Tags
건너뛰기...
이 컬렉션의 비디오:

Now Playing
항온 풍속 측정: 난류 경계층 흐름 연구 도구
Aeronautical Engineering
7.2K Views

모형 항공기의 공기 역학적 성능: DC-6B
Aeronautical Engineering
8.3K Views

프로펠러 특성: 성능 관련 피치, 직경 및 블레이드 수의 변화
Aeronautical Engineering
26.4K Views

에어포일 동작: Clark Y-14 날개의 압력 분포
Aeronautical Engineering
21.2K Views

Clark Y-14 날개 성능: 고양력 장치 (플랩 및 슬랫) 적용
Aeronautical Engineering
13.4K Views

난류 구체 방식: 풍동 흐름 품질 평가
Aeronautical Engineering
8.7K Views

교차 원통형 흐름: 압력 분포 측정 및 항력 계수 추정
Aeronautical Engineering
16.2K Views

노즐 분석: 수렴 및 수렴 전달 노즐에 따른 마하수 및 압력의 변화
Aeronautical Engineering
37.9K Views

슐리렌 이미징: 초음속 흐름 특징을 시각화하는 기술
Aeronautical Engineering
11.7K Views

회류 수조 흐름 시각화: 델타 날개 위 첨단 소용돌이 관찰
Aeronautical Engineering
8.1K Views

표면 염료 흐름 시각화: 초음속 흐름 내 흐름맥 패턴을 관찰하는 정성적 방법
Aeronautical Engineering
4.9K Views

피트 정압관: 풍량 측정 장치
Aeronautical Engineering
49.1K Views

압력 변환기: 피트 정압관을 사용한 보정
Aeronautical Engineering
8.5K Views

실시간 비행 제어: 임베디드 센서 교정 및 데이터 수집
Aeronautical Engineering
10.3K Views

멀티콥터 공기역학: 헥사콥터 추력 특성화
Aeronautical Engineering
9.1K Views
Copyright © 2025 MyJoVE Corporation. 판권 소유