Molecular Orbital (MO) Theory
Source: Tamara M. Powers, Department of Chemistry, Texas A&M University
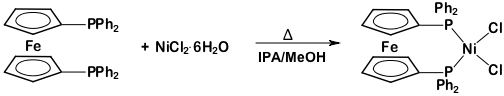
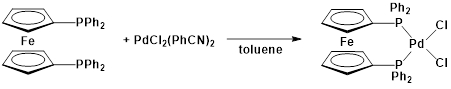
This protocol serves as a guide in the synthesis of two metal complexes featuring the ligand 1,1'-bis(diphenylphosphino)ferrocene (dppf): M(dppf)Cl2, where M = Ni or Pd. While both of these transition metal complexes are 4-coordinate, they exhibit different geometries at the metal center. Using molecular orbital (MO) theory in conjunction with 1H NMR and Evans method, we will determine the geometry of these two compounds.
NOTE: For safety precautions, the Schlenk line safety should be reviewed prior to conducting the experiments. Glassware should be inspected for star cracks before using. Care should be taken to ensure that O2 is not condensed in the Schlenk line trap if using liquid N2. At liquid N2 temperature, O2 condenses and is explosive in the presence of organic solvents. If it is suspected that O2 has been condensed or a blue liquid is observed in the cold trap, leave the trap
Pd(dppf)Cl2:
1H NMR (chloroform-d, 400 MHz, δ, ppm): 4.22 (alpha-H), 4.42 (beta-H), 7.89, 7.44, 7.54 (aromatic)3.
Ni(dppf)Cl2:
1H NMR (chloroform-d, 300 MHz, δ, ppm): 20.85, 10.04, 4.23, 3.98, 1.52, -3.31, -7.10.
Evans Method, looking at the 19F shift of trifluorotoluene:
Log in or to access full content. Learn more about your institution’s access to JoVE content here
This video demonstrated how MO theory can be used as a model of bonding in transition metal complexes. We synthesized two complexes with the general formula M(dppf)Cl2. When M = Ni, the 4-coordinate complex exhibits a tetrahedral geometry. Replacing the Ni atom with a larger transition metal (Pd), the molecule takes on square planar geometry.
Previously, we learned about the important role ferrocene plays in the field of organometallic chemistry. Substituted ferrocenes, including dp
- Corain, B., Longato, B., Favero, G. Heteropolymetallic Complexes of 1,1’-Bis(diphenylphosphino)ferrocene (dppf). III*. Comparative Physicochemical Properties of (dppf)MCl2 (M = Co, Ni, Pd, Pt, Zn, Cd, Hg). Inorg Chim Acta. 157, 259-266 (1989).
- Cullen, W. R., Einstein, F. W. B., Jones, T., Kim, T.-J. Structures of three hydrogenation catalysts [(P-P)Rh(NBD)]ClO4 and some comparative rate studies where (P-P) = (η5-R1R2PC5H4)(η5-R3R4PC5H4)Fe (R1 = R2 = R3 = R4 = Ph, R1 = R2 = Ph, R3 = R4 = CMe3, R1 = R3 = Ph, R2 = R4 = CMe3). Organometallics. 4(2), 346-351 (1983).
- Colacot, T. J., C.-Olivares, R., H.-Ortega, S. Synthesis, X-ray, spectroscopic and a preliminary Suzuki coupling screening studies of a complete series of dppfMX2 (M = Pt, Pd, X = Cl, Br, I). J Organomet Chem. 637-639, 691-697 (2001).
- Rudie, A. W., Lichtenberg, D. W., Katcher, M. L., Davison, A. Comparative Study of 1,1’-bis(diphenylphosphino)cobaltocinium hexafluorophosphate and 1,1’-bis(dipenylphosphino)ferrocene as Bidentate Ligands. Inorg Chem. 17(10), 2859-2863, 1978.
Skip to...
Videos from this collection:
Now Playing
Molecular Orbital (MO) Theory
Inorganic Chemistry
34.5K Views
Synthesis Of A Ti(III) Metallocene Using Schlenk Line Technique
Inorganic Chemistry
30.7K Views
Glovebox and Impurity Sensors
Inorganic Chemistry
18.3K Views
Purification of Ferrocene by Sublimation
Inorganic Chemistry
53.4K Views
The Evans Method
Inorganic Chemistry
65.8K Views
Single Crystal and Powder X-ray Diffraction
Inorganic Chemistry
101.2K Views
Electron Paramagnetic Resonance (EPR) Spectroscopy
Inorganic Chemistry
25.0K Views
Mössbauer Spectroscopy
Inorganic Chemistry
21.7K Views
Lewis Acid-Base Interaction in Ph<sub>3</sub>P-BH<sub>3</sub>
Inorganic Chemistry
37.6K Views
Structure Of Ferrocene
Inorganic Chemistry
77.7K Views
Application of Group Theory to IR Spectroscopy
Inorganic Chemistry
44.1K Views
Quadruply Metal-Metal Bonded Paddlewheels
Inorganic Chemistry
14.8K Views
Dye-sensitized Solar Cells
Inorganic Chemistry
15.1K Views
Synthesis of an Oxygen-Carrying Cobalt(II) Complex
Inorganic Chemistry
50.0K Views
Photochemical Initiation Of Radical Polymerization Reactions
Inorganic Chemistry
16.5K Views
ABOUT JoVE
Copyright © 2025 MyJoVE Corporation. All rights reserved