1. 热线系统动态响应确定

此过程的目的是了解测能仪系统对流量信号变化的响应速度。通过应用方波来测量信号打开和关闭时的频率响应，可以衡量此功能。

1. 使用支撑轴将 CTA 系统的热线探头固定在风洞内。
2. 设置直流电源、信号发生器和示波器，并连接它们，如图 2（a）所示。信号发生器为惠斯通桥提供方波输入，输出波形在示波器上可视化。
3. 打开热线电源、示波器和信号发生器。
4. 设置信号发生器以输出具有 150 mV 振幅和 10 kHz 频率的方波。
5. 观察示波器中的输出信号，确保输出波形频率和振幅正确。
6. 关闭测试部分并插入串行端口。打开风洞，将空速设置为 40 mph。
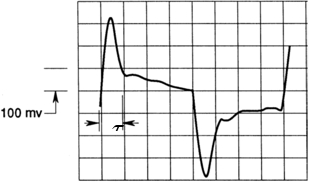
7. 一旦气流稳定下来，从示波器测量信号过冲的宽度。"有关*的定义，请参阅图 3。
8. 使用*的测量值使用公式获得热线系统的截止频率：f_切= 1/1.5°。
9. 关闭风洞。

2. 热线校准

本程序的目的是建立惠斯通桥的空速和电势之间的相关性。这允许测量流速。

1. 将热线探头调整到垂直位置，使其与平板的距离足够远，在这种情况下，这是风洞的地板，因此它位于自由流区域。
2. 启动风洞控制软件。
3. 打开虚拟仪器软件，将采样频率设置为 10 kHz，将采样数设置为 100，000。这些参数由要测量的流场的流量特征决定，并且可能因目标统计信息的收敛要求知识而异。
4. 将风洞速度设置为 0 mph，并记录惠斯通桥上的电压。
5. 将风洞空气速度以3英里/小时为增量增加到15英里/小时。在测量电压之前，让流量在每个空气速度下稳定下来。
6. 将风洞空气速度以 5 mph 的增量增加到 60 mph，并在每个增量处测量电压。
7. 完成所有测量后，将气流降至 30 mph，然后关闭风洞。

图 3.信号过冲宽度的原理图，如在方波测试期间在示波器上观察到的。

3. 边界层测量

1. 使用与上一实验部分相同的设置，缓慢降低热线探头，直到它接触测试部分地板，该测试部分将充当平板。
2. 打开风洞，将空速设置为 40 mph，采样频率为 10 kHz，将采样数量设置为 100，000，与之前一样。
3. 在最低价垂直设置（位于平板旁边和边界层中）记录电压读数。
4. 垂直移动探头，以 0.05 mm 的步长将高度增加到 0.50 mm 的高度，并在垂直位置记录电压读数。
5. 将探头高度以 0.10 mm 的步长增加到 1.50 mm 的高度，并将电压读数记录在垂直位置。
6. 以 0.25 mm 的步长将探头高度增加到 4.00 mm 的最终高度，并在垂直位置记录电压读数。
7. 完成所有测量后，将风速降至 20 mph，然后关闭风洞、CTA、电源、示波器和功能发生器。