24.14 : Electrostatic Boundary Conditions
Consider an external electric field propagating through a homogeneous medium. When the electric field crosses the surface boundary of the medium, it undergoes a discontinuity. The electric field can be resolved into normal and tangential components. The amount by which the field changes at any boundary is given by the difference between the field components above and below the surface boundary.
The surface integral of an electric field is given by Gauss's law in integral form and is related to the total enclosed charge. Consider a Gaussian pillbox on the surface boundary. The surface integral of the field is the sum of the integrals over all the surfaces of the pillbox. If the thickness of the pillbox tends to zero, then the surface integral includes only the contributions of the pillbox faces above and below the boundary. Solving this gives the discontinuity of the normal component of the electric field at any surface boundary.
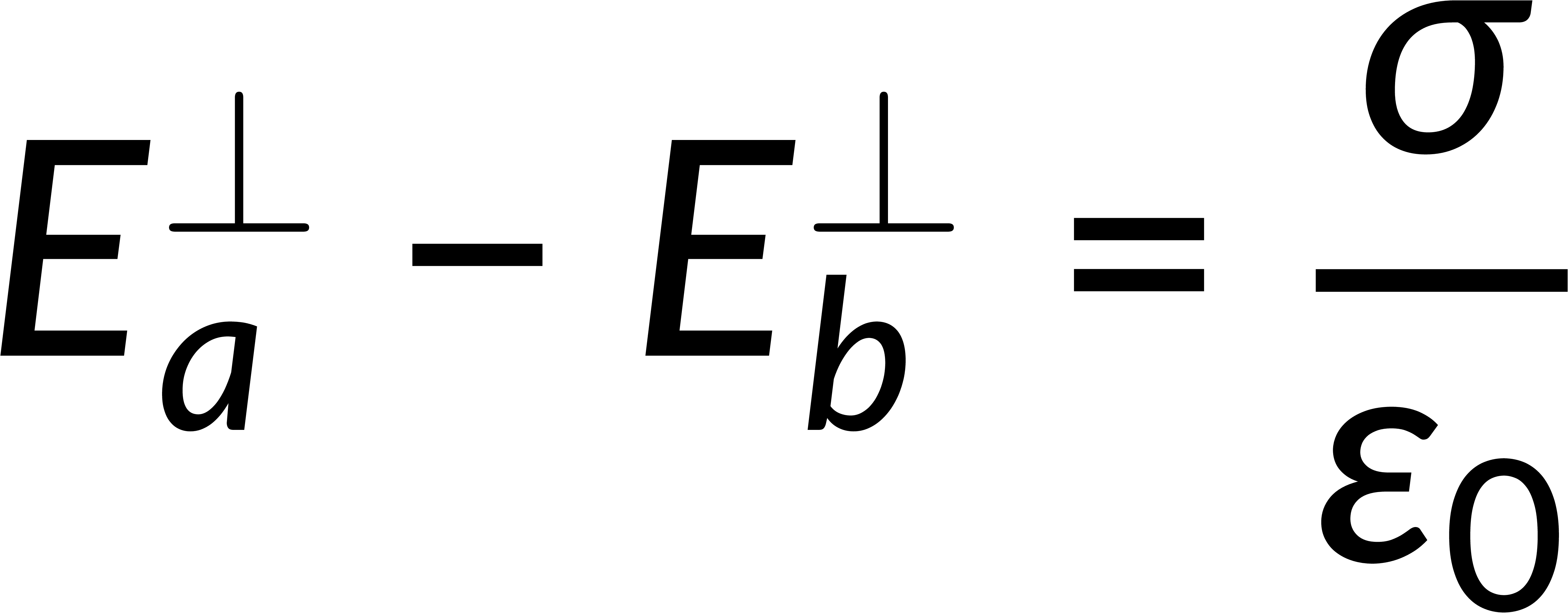
To estimate the discontinuity of the tangential component, consider a loop on the surface boundary. The integration of the electric field over this closed path is zero. Breaking this into the contributions from four parts of the loop and applying the condition that the thickness of the loop tends to zero implies that the tangential component of the electric field is continuous across the boundary.
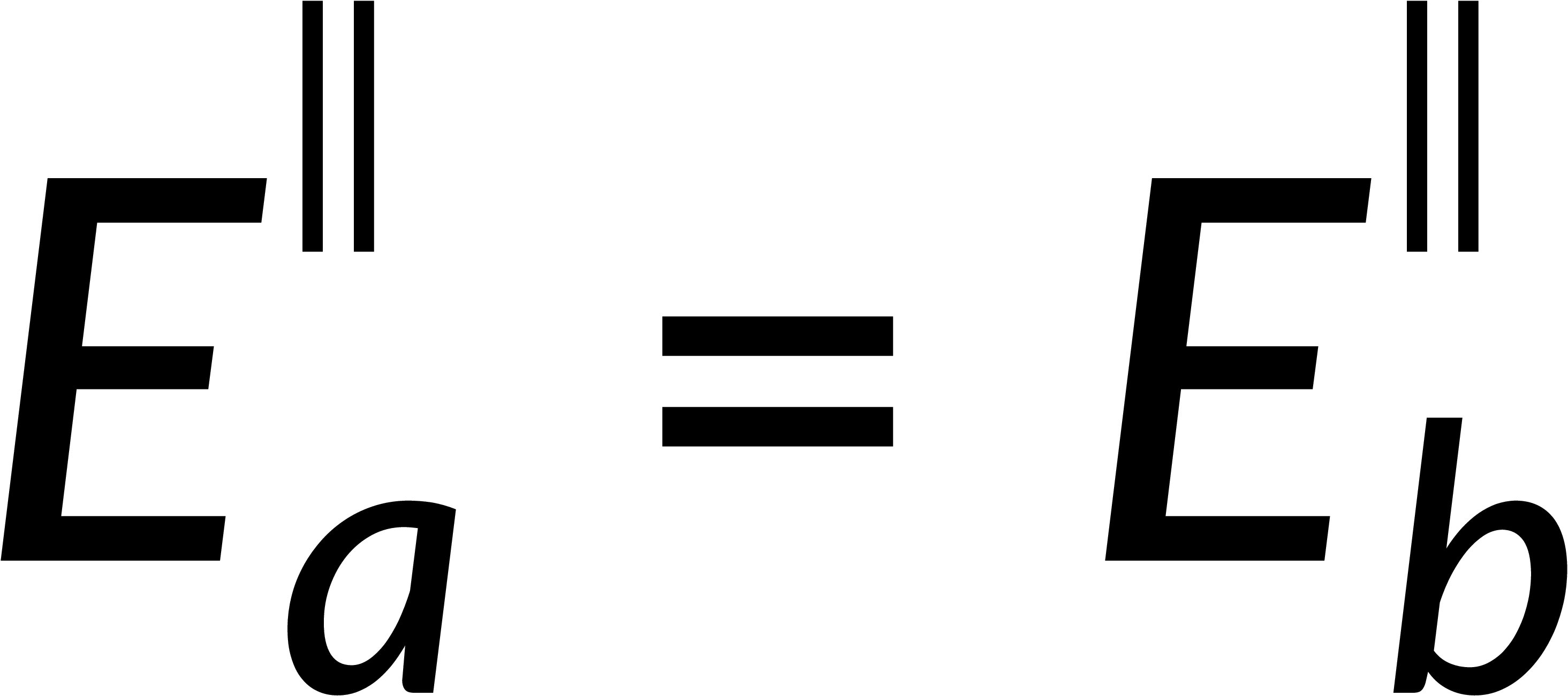
Combining the normal and tangential equations, the field at the boundary can be written by defining the unit vector perpendicular to the surface. It is known that the electric field is the negative gradient of the potential. The line integral of the field from below to above the boundary tends to zero, implying that the potential is continuous across any boundary.
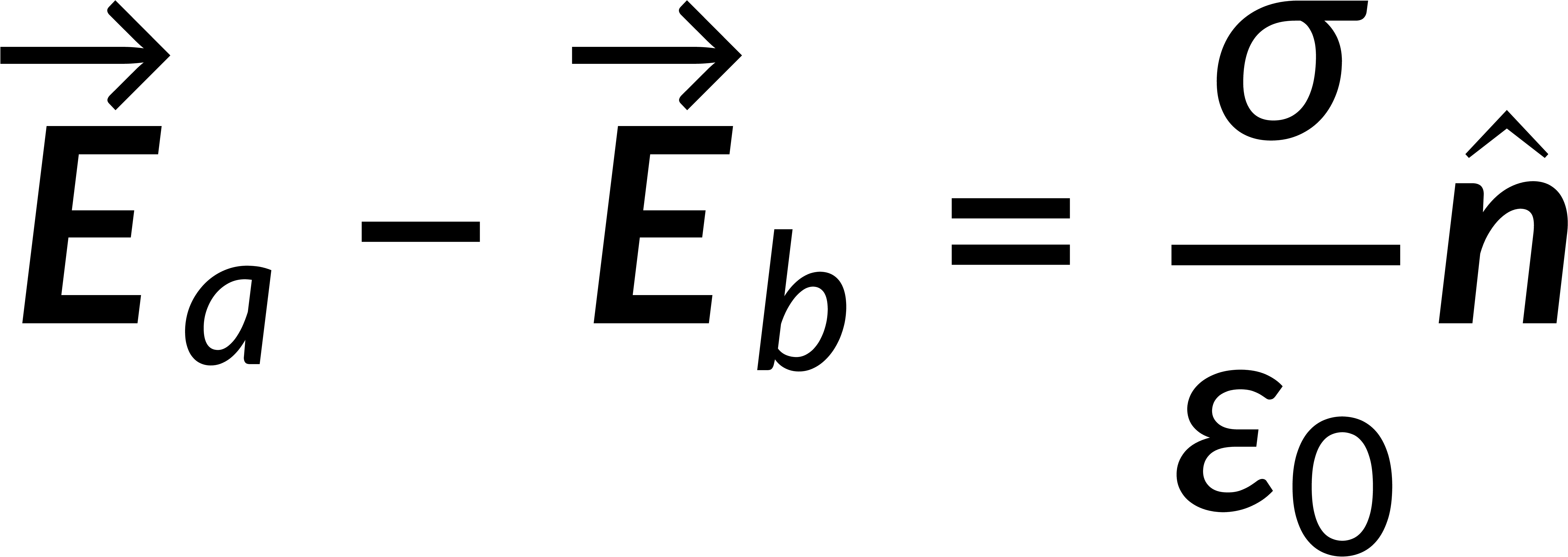
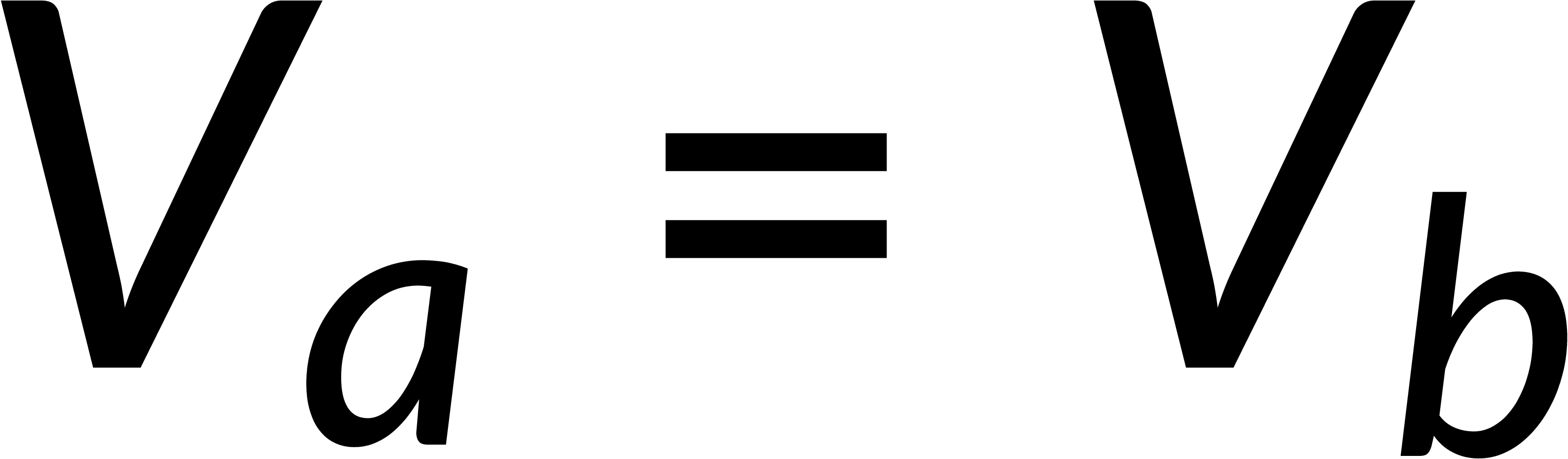
From Chapter 24:

Now Playing
24.14 : Electrostatic Boundary Conditions
Electric Potential
420 Views

24.1 : אנרגיה פוטנציאלית חשמלית
Electric Potential
5.7K Views

24.2 : אנרגיה פוטנציאלית חשמלית בשדה חשמלי אחיד
Electric Potential
4.6K Views

24.3 : אנרגיה פוטנציאלית חשמלית של שני מטענים נקודתיים
Electric Potential
4.5K Views

24.4 : פוטנציאל חשמלי והפרש פוטנציאלים
Electric Potential
4.3K Views

24.5 : מציאת פוטנציאל חשמלי משדה חשמלי
Electric Potential
4.0K Views

24.6 : חישובי פוטנציאל חשמלי I
Electric Potential
1.9K Views

24.7 : חישובי פוטנציאל חשמלי II
Electric Potential
1.6K Views

24.8 : משטחים וקווי שדה שווי פוטנציאל
Electric Potential
3.6K Views

24.9 : משטחים ומוליכים שווי פוטנציאל
Electric Potential
3.3K Views

24.10 : קביעת שדה חשמלי מפוטנציאל חשמלי
Electric Potential
4.4K Views

24.11 : המשוואה של פואסון ולפלס
Electric Potential
2.6K Views

24.12 : ואן דה גראף גנרטור
Electric Potential
1.7K Views

24.13 : אנרגיה המשויכת לחלוקת מטען
Electric Potential
1.5K Views

24.15 : משפט הייחוד השני
Electric Potential
979 Views
Copyright © 2025 MyJoVE Corporation. All rights reserved