In electrostatics, the electric field can be written as the negative gradient of the potential. In magnetostatics, the zero divergence of the magnetic field ensures that the magnetic field can be expressed as the curl of a vector potential. This potential is known as the magnetic vector potential.
Consider an ideal solenoid with n turns per unit length and radius R. If I is the current through the solenoid, the magnetic field inside the solenoid is expressed as the product of vacuum permeability, the number of turns per unit length, and the current. Conversely, the magnetic field outside the solenoid is zero. Considering this, what is the vector potential for an ideal solenoid?
The magnetic flux through the solenoid is given by
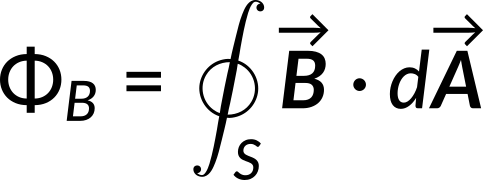
Since the magnetic field equals the curl of the vector potential, the magnetic flux can be rewritten in terms of the vector potential.
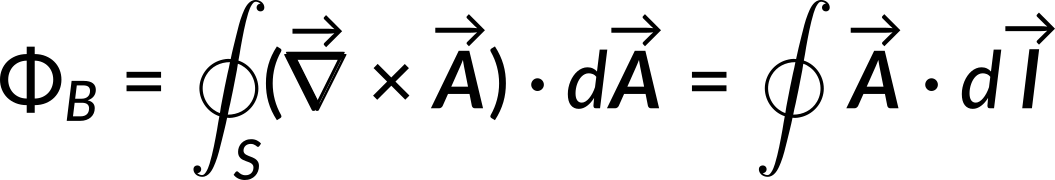
Thus, the line integral of the magnetic vector potential equals the surface integral of the magnetic field.
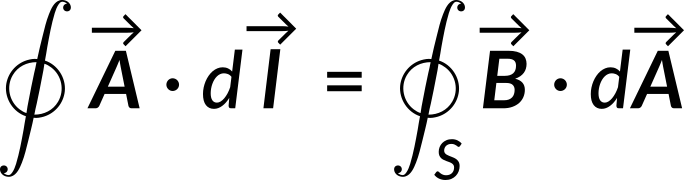
Now consider a circular Amperian loop of radius r inside the solenoid. The magnetic flux through this loop is given by
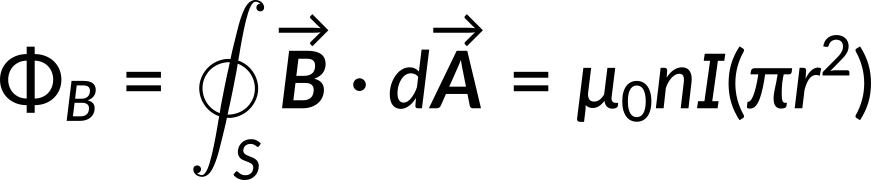
Equating the magnetic flux to the line integral of the magnetic vector potential, the expression for the vector potential can be obtained.
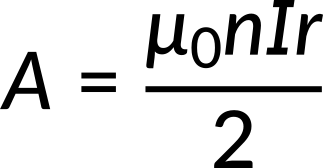
The vector potential mimics the magnetic field and acts along the circumference.
Do Capítulo 29:

Now Playing
29.14 : Potencial Vetorial Magnético
Fontes de Campos Magnéticos
443 Visualizações

29.1 : Campo Magnético Gerado por Cargas em Movimento
Fontes de Campos Magnéticos
8.0K Visualizações

29.2 : Lei de Biot-Savart
Fontes de Campos Magnéticos
5.5K Visualizações

29.3 : Lei de Biot-Savart: Resolução de Problemas
Fontes de Campos Magnéticos
2.2K Visualizações

29.4 : Campo Magnético Gerado por um Fio Retilíneo Fino
Fontes de Campos Magnéticos
4.5K Visualizações

29.5 : Campo Magnético Devido a Dois Fios Retos
Fontes de Campos Magnéticos
2.2K Visualizações

29.6 : Força Magnética Entre Duas Correntes Paralelas
Fontes de Campos Magnéticos
3.3K Visualizações

29.7 : Campo Magnético em uma Espira de Corrente
Fontes de Campos Magnéticos
4.1K Visualizações

29.8 : Divergência e Rotacional do Campo Magnético
Fontes de Campos Magnéticos
2.6K Visualizações

29.9 : Lei de Ampère
Fontes de Campos Magnéticos
3.5K Visualizações

29.10 : Lei de Ampère: Resolução de Problemas
Fontes de Campos Magnéticos
3.4K Visualizações

29.11 : Solenóides
Fontes de Campos Magnéticos
2.4K Visualizações

29.12 : Campo Magnético de um Solenóide
Fontes de Campos Magnéticos
3.5K Visualizações

29.13 : Toróides
Fontes de Campos Magnéticos
2.7K Visualizações

29.15 : Potencial Devido a um Objeto Magnetizado
Fontes de Campos Magnéticos
225 Visualizações
See More
Copyright © 2025 MyJoVE Corporation. Todos os direitos reservados