قياس صلابة الكمي المجهزة AFM-تسنن

Summary

This experimental protocol describes how atomic force microscopy can be used to measure hardness at the true nanometer scale and to detect single atomistic plasticity events.

Abstract

In this work, a combination of amplitude-modulated non-contact atomic force microscopy and atomic force spectroscopy is applied for instrumented hardness measurements on an Au(111) surface with atomistic resolution of single plasticity events. A careful experimental procedure is described that includes the force sensor selection, its calibration, the calibration of the cantilever deflection detection system, and the minimization of instrumental drift for accurate and reproducible force-distance measurements. Also, a method for the data analysis is presented that allows the extraction of force-penetration curves from recorded force-distance curves. A typical curve displays a clear elastic deformation regime up to the first plasticity event, or pop-in, with a length in the range of one to two Burger's vectors. Later plasticity events exhibit the same magnitude. The work of plasticity is further extracted from the measurements. Finally, the hardness is determined in combination with the indentation curve using non-contact atomic force microscopy images of the remaining indents.

Introduction

For the last 150 years, hardness values have been used to characterize the mechanical behavior of materials and to predict their performance under various loading conditions. The hardness of a material describes the resistance of its surface to the penetration of a harder indenter. For metallic materials, the hardness relates to resistance to plastic flow.

Although the implementation of hardness testing has proven to be relatively easy, the yielded results have long been rather empirical, making them unable to describe the intrinsic properties of the investigated material. The empirical nature of hardness testing results has been a consequence of the use of various indenter geometries, each of which has a different established hardness scale. However, all empirical hardness scales, such as the Brinell hardness (HB) test for spherical indenters and the Vickers (HV) and Knoop (HK) hardness tests for different types of four-sided pyramids, have one thing in common: the hardness number is determined from the ratio of the applied load to the developed area of the remaining indent. In an attempt to relate the hardness of metals to their fundamental mechanical properties, the hardness measured with a spherical indenter has been defined as the ratio of the applied load to the projected area of the remaining indent. This hardness value, also known as the Meyer's hardness (H), is equal to the mean contact pressure and directly relates to the yield strength of non-work-hardening metals¹.

Hardness testing has long been limited to the macro-scale, in which case the sizes of indents have been measured by optical means. The development of instrumented indentation, where force-displacement curves are recorded, has been recognized as a valuable alternative for determining the hardness of a material, although the size of the remaining indent may be too small to be accurately imaged. In this case, the projected area of the indent is calculated from the tip displacement according to a so-called indenter-area function². Due to this development, the analysis of the curvature of force-displacement curves and of the occurrence of distinct plasticity events in the shape of pop-ins is now widely used to study the mechanisms of plastic deformation at the micrometer scale, such as by means of nanoindentation³.

It is well accepted that the carriers of plastic deformation in metals, i.e., dislocations, operate at the nanometer scale. To understand their modes of operation at the atomic level, new experimental techniques with atomic resolution are needed. Initial investigations of atomistic plasticity events at the interfaces between nanometer-sized single asperities and single crystalline Au surfaces of different orientations have been carried out by interfacial force microscopy (IFM)^{4, 5}. Atomic force microscopy (AFM) indentation has been applied to observe the nucleation and gliding of single dislocations in KBr(100) single crystals⁶, Cu(100)⁷, and Au(111)^{8, 9}. There, atomistic plasticity events were observed in the shape of pop-ins, with lengths in the range of 1 Å. AFM indentation has also been used to measure the nano-hardness and -elasticity of gold nano-island grown on mica¹⁰. In this work, the nano-hardness was found to be smaller than the bulk value and was observed to depend linearly on the indentation area. Also, a detailed measurement protocol has been proposed to determine the hardness of hard surfaces, such as fused quartz and silicon, by AFM indentation with a diamond-tipped sapphire cantilever¹¹. In particular, this method takes into account the non-linearity of the photo-sensitive deflection detectors for large cantilever deflection¹². More recently, AFM indentation and non-contact AFM imaging have been combined to quantitatively determine the hardness and the fundamental mechanisms of plastic deformation of a Pt(111) single crystalline surface and Pt-based metallic glass¹³. For Pt(111), plastic deformation mechanisms at the nanometer scale were found to be consistent with the discrete mechanisms established for larger scales. Further, the nanometer-scale plastic deformation of the metallic glass was found to be not discrete, but rather continuous and highly localized around the indenting tip. These results revealed a lower size limit for metallic glasses, below which shear transformation mechanisms are not activated by indentation. AFM indentation has also been used to determine the hardness values of biological samples (such as collagen fibrils)¹⁴, polymers¹⁵, and colloidal crystals¹⁶.

In the present work, a careful experimental procedure is described that includes the force sensor selection, its calibration, the calibration of the cantilever deflection detection system, and the minimization of instrumental drift for accurate and reproducible force-distance measurements. Also, a method for the data analysis is presented that allows the extraction of force-penetration curves from recorded force-distance curves. Further representative results are shown and discussed in the light of recent findings in the field of plastic deformation of small volumes.

For this experiment, an atomic force microscope (AFM) was used. The cornerstone of an AFM is its micro-fabricated force sensor (usually a cantilever beam), with a sharp tip at the end whose radius is in the range of several nanometers. In the particular configuration of the instrument used for this experiment, the cantilever is mounted onto a piezo-electric z-scanner, while the sample to be investigated is mounted onto a piezo-electric x/y-scanner. During AFM imaging, the force sensor tip is scanned over a sample to register interaction forces with the sample surface that result in a deflection of the cantilever according to Hooke's law. The static or dynamic deflection of the cantilever can be measured with an optical beam deflection detector, consisting of a laser diode, a set of mirrors, and a photodiode that converts the cantilever deflection into a voltage. During imaging, the signal at the photodiode is controlled by a feedback loop so as to keep the interaction forces at the sample surface; this results in adjustments of the z-scanner position, which are recorded and displayed as a topography image.

Prerequisites for the experiment described below are that the piezo-electric scanners of the atomic force microscope are well-calibrated and that the instrument stays in the laboratory at a constant temperature and humidity level. The reader should be aware that, depending on the atomic force microscope model, some of the experimental procedure steps may have to be modified. In particular, all measurements are performed after setting the scanners' ranges to "small"; this function allows for the reduction of the x/y-scanner range to 5 x 5 µm² and the z-scanner range to 4 µm, which ensures a resolution at small scales. This function is not available on all commercial AFM and is not mentioned in the remainder of the text.

For data analysis, the use of the free SPM data analysis software Gwyddion¹⁷ and the Matlab software package¹⁸ are recommended.

This protocol gives a description of the experimental procedure to be followed in order to perform instrumented hardness measurements by AFM. Since the handling of different commercial AFMs may differ from one model to the other, the reader should refer to the manual provided by the AFM manufacturer for detailed setting procedures and software information. In the following text, in order to reproduce the described experiment, it is assumed that the reader is familiar with the handling of the particular AFM used here.

Protocol

1. دور فعال مجموعة المتابعة والمعايرة

1. دور فعال مجموعة المتابعة
   1. استخدام الكابولي المغلفة الماس صلب من نوع DT-NCLR أو CDT-NCLR مع أول تردد صدى مجانا و _0،1 ≥ 180 كيلو هرتز، وهو عامل الجودة س ≥ 300، والانحناء صلابة ك ≥ 40 نيوتن / متر.
   2. جبل ناتئ المحددة إلى حامل لقط المقدمة من قبل الشركة المصنعة فؤاد. عناية خاصة لوضع ناتئ بحيث محورها الطويل هو عمودي على اتجاه المسح السريع للفؤاد. بدلا من ذلك، الغراء ناتئ على حامل ناتئ المقدمة من قبل الشركة المصنعة AFM باستخدام الغراء الايبوكسي المزدوج المكون.
   3. جبل حامل ناتئ على رأس فؤاد واستخدام المجهر الضوئي المتوفرة عادة مع نظام AFM للتركيز على ناتئ فؤاد. الاختيار المزدوج المحور ناتئ الطويل هو عمودي على اتجاه المسح السريع. إن لم يكن، والعودة إلىالقسم 1.1.2.
   4. محاذاة شعاع الليزر بحيث ينعكس ذلك في نهاية ناتئ. رصد مبلغ الجهد في الثنائي الضوئي وإجراء الضبط الدقيق لتعظيم إشارة المبلغ. القيم إشارة مبلغ النموذجية هي في حدود 2 V.
   5. ضبط زوايا الميل الأفقي والرأسي في المرآة ذلك لتحقيق بقعة الليزر ينعكس في وسط الضوئي، حيث الفولتية المقابلة إلى تشريد الرأسي والأفقي ما يقرب من الصفر.
2. معايرة
   1. إجراء مسح تردد لتحديد أول الانحناء صدى مجانا و _0،1 للتعزية.
   2. تحديد صلابة الانحناء للك ناتئ، وتحسب وفقا ^ل19
      (1)
      حيث E هو معامل يونغ، L هو طول ناتئ، ث هو عرض cantilمن أي وقت مضى، ور هو سمك. تحقيقا لهذه الغاية، وقياس الطول والعرض للناتئ بواسطة المجهر الضوئي أو المجهر الإلكتروني لتحسين دقة. حساب سمك ناتئ من أول تردد الحرة الانحناء صدى لها و _0،1، وفقا ل
      (2)
      حيث ρ هي كثافة الكتلة.
   3. حدد القيمة الافتراضية لحساسية الضوئي لنوع ناتئ معين ليتم استخدامها للتجربة في إعداد القائمة من فؤاد. جلب رأس ناتئ في اتصال مع العينة المرجعية في F الحمل _ن = 10 ن ن عن طريق النقر على زر النهج.
   4. فتح القائمة قوة التحليل الطيفي في البرنامج AFM وتعيين التراجع النسبي وتمديد ض الماسح الضوئي إلى 50 نانومتر، وض الماسح الضوئي تراجع / تمديد إلى 0.3 ميكرون / ثانية. بذلك، تسجيل منحنى القوة لمسافات سوفتتكون أولا من تراجع للض الماسح الضوئي إلى 50 نانومتر بعيدا عن سطح العينة وبعد ذلك سلسلة من النهج والتراجع عن مسافة واحدة.
   5. تسجيل منحنى المسافة القوة مع المعايير المحددة المقترحة في 1.2.4 على سطح أملس وغير المتوافقة، مثل الماس النانو بلورية أو الياقوت، وذلك لتجنب الآثار تشوه العينة. للقيام بذلك انقر على زر تحصل عليها في القائمة قوة التحليل الطيفي للبرمجيات فؤاد.
   6. تناسب جزء مثير للاشمئزاز من منحنى القوة لمسافات مع وظيفة خطية، في القائمة معايرة البرمجيات فؤاد. المنحدر معكوس الخط المناسب يتوافق مع حساسية الضوئي S. استبدال قيمة العزم على القيمة الافتراضية من البرنامج أداة في القائمة معايرة البرنامج AFM بالضغط على زر معايرة تنفيذ.

إعداد 2. عينة

ملاحظة: عينة تقاس في ثيتتكون الصورة التجربة من 100 نانومتر سميكة، على نحو سلس بالذرة الاتحاد الافريقي (111) رقيقة نمت على الميكا بواسطة ترسيب البخار المادي.

1. جبل العينة على صاحب العينة المغناطيسي المقدمة من قبل الشركة المصنعة الصك عن طريق الوجهين الشريط الكربون. من أجل تجنب الانجراف من العينة خلال القياسات، جبل العينة قبل يوم واحد من القياسات، وذلك للسماح الشريط الكربون الاسترخاء. بدلا من ذلك، جبل العينة على حامل مع طلاء الفضة، الذي يجف عادة في غضون بضع دقائق.
2. جبل صاحب العينة المغناطيسي على الماسح الضوئي س / ص.

3. إجراء القياس

1. ضبط وتيرة التذبذب قليلا خارج الرنين (في هذه التجربة و = 190.67 كيلو هرتز) والسعة التذبذب في A = 20 نانومتر ملاحظة أن هذه القيم يتم تعيين تلقائيا من قبل البرنامج أداة لهذا ناتئ معين. تعيين نقطة مجموعة التذبذب يدويا في _{مجموعة نقطة} = 5 نانومتر.
2. رسمناتئ نحو سطح العينة باستخدام الخطوة الحركية للفؤاد. تأكد من أن أجهزة الاستشعار القوة لا تتصادم مع سطح العينة. الحفاظ على ناتئ في التركيز خلال نهج الخشنة ووقف نهج الخشن قبل سطح العينة هو في التركيز المثالي.
3. نهج تلقائيا استشعار القوة من خلال النقر على زر النهج. مرة واحدة سعة التذبذب وصلت نقطة مجموعتها، وغيض على استعداد لفحص تضاريس سطح العينة.
4. تسجيل سلسلة من الصور الطوبوغرافية في مجالات تتراوح بين 5 × 5-1 × 1 μm² (إن وجدت، وضبط المنحدر من إشارة التضاريس عن طريق إمالة س / ص الماسح الضوئي). تأكد من أن الصور المتتالية لنفس المنطقة لا يحمل أي علامة من الانجراف وأن موقف ض الماسح الضوئي يبقى ثابتا تقريبا. إذا لم تكن هذه هي الحالة، يستمر التصوير حتى استقر النظام.
5. مرة واحدة وقد استقر النظام وتم العثور على نحو سلس منطقة μm² 1 × 1، التراجع عن وأورثي استشعار بضعة ميكرومتر من سطح العينة عن طريق النقر على زر تتراجع.
6. تحديد وضع قوة التحليل الطيفي في القائمة أداة ونقل استشعار القوة لمنتصف المحددة مسبقا في منطقة μm² 1 × 1، مع قوة وضع نقطة من 10 نانومتر. رصد موقف ض الماسحة الضوئية إلى أن يبقى ثابتا.
7. حدد الشبكة 2 × 2 نقاط الذي يتوافق مع مركز المحددة مسبقا في منطقة μm² 1 × 1 المركز. تعيين المسافة بين نقطتين المجاورة المقبلة في 500 نانومتر.
8. تعيين المسافة الماسح الضوئي النسبية تختلف 0-150 نانومتر بسرعة 300 نانومتر / ثانية ومن ثم التراجع على مسافة واحدة وبنفس السرعة. ونظرا لزاوية الميل للناتئ فيما يتعلق سطح العينة، وتطبيق تصحيح الميل عن طريق تحريك الماسح الضوئي الجانبي بواسطة Z × تان φ خلال عمودي Z تمديد الماسح الضوئي، حيث φ هي زاوية الميل ^20.
   ملاحظة: هناك عدد قليل من معاهدحساب ruments للإمالة ناتئ في التحليل الطيفي قوتها أو وضع المسافة البادئة. وهذا هو الحال بالنسبة لفؤاد المستخدمة في هذا العمل.
9. اضغط على زر البدء في البرنامج أداة لبدء الحصول على البيانات AFM المسافة البادئة.
10. وبمجرد الانتهاء من قياسات AFM المسافة البادئة، التراجع عن استشعار القوة بضعة ميكرومتر بعيدا عن سطح العينة.
11. حدد عدم الاتصال وضع فؤاد التصوير في القائمة أداة البرمجيات وكرر الإجراء الموضح في الأقسام 3.1 و 3.2.
12. إجراء فحص على نفس 1 × 1 مساحة μm² كما في القسم 3.3 وذلك لتحديد الموضع الدقيق من المسافات البادئة. مسح سطح المزيد على مساحة nm² 500 × 500 لا يمكن أن يؤديها لصورة تزكي المتبقية بمزيد من التفصيل.

تحليل 4. البيانات

1. معالجة الصورة
   1. معالجة الصور تضاريس سجلت حتى محاذاة خطوط في دير مسح سريعection على أساس الفرق المتوسط. استخدام دالة مضمنة من Gwyddion.
2. حساب مساحة المسقط _p من المسافات البادئة باستخدام وظيفة تحليل المسافة البادئة Gwyddion.
3. تقدير شكل AFM غيض من الصور تضاريس المسافات البادئة باستخدام وظيفة تحليل غيض من Gwyddion. ثم المتوسط الصور شكل قمة وقياس نصف افتتاح الزاوية α في شكل قمة المتوسط.
4. تحويل منحنيات القوة لمسافات إلى منحنيات قوة النزوح عن طريق حساب تشريد طرف δ وفقا ^ل13
   (3)
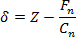
   حيث Z هو موقف الماسح الضوئي النسبي.
5. الآن، رسم القوة مقابل تشريد طرف. منحنى الناتج عادة يعرض ما يسمى البوب ​​الإضافية، مع أطوال في حدود عدة 100 م، التي تتوافق مع أحداث اللدونة ذري. استخدم لأول مرة من تساالبريد البوب الإضافية لتحديد تشريد طرف في حدود مرنة δ _شرم ^4.
6. تناسب الجزء المرن من منحنى القوة التشريد مع وظيفة الهرتزية ^21.
   (4)
   حيث R هو نصف قطرها طرف وE ^'* هو انخفاض معامل المرونة، التي قدمها ، مع M _{ق، ر} كونها معامل المسافة البادئة من العينة ومن طرف، على التوالي. في هذه الحالة، المعلمة صالحة ل .
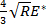7. تمديد وظيفة مناسبة في نظام اللدونة وذلك لحساب عمل اللدونة W _{اللدونة} من الفرق المساحية بين وظيفة مناسبة ومنحنى التجريبية ^21.
8. حساب صلابة من العينة وفقا ^{ل1، 2}
   (5)
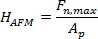
   و
   (6)
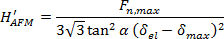
   حيث F _{ن، الحد الأقصى} هو الحمل القصوى المطبقة، _ف هي المنطقة المتوقعة من المسافة البادئة المحسوبة في القسم 4.2، α هي الزاوية الشوط الأول للطرف المحسوبة في القسم 4.3، δ _ايل هو النزوح طرف في اللدونة الأولى الحدث، وδ _{الحد الأقصى} هو تشريد طرف القصوى (انظر القسم 4.4).

النتائج

في هذا العمل، تم احتساب صلابة الانحناء للك ناتئ وفقا لنظرية شعاع الهندسية ^19. لمعين ناتئ المغلفة الماس المستخدمة في هذا العمل، وجدنا ك = 55.69 نيوتن / متر. لاحظ أننا أهملنا طلاء الماس. سمك الطلاء الماس 1-2 أوامر من حجم أصغر من سمك ناتئ، وبال?...

Discussion

تم عرض طريقة لإجراء سلسلة من الفتحات على الاتحاد الافريقي (111) سطح الأغشية الرقيقة مع AFM طرف الماس المغلفة. أجريت عدم الاتصال التصوير فؤاد وفؤاد تسنن مع استشعار قوة نفسه. متطلبات التصوير عدم الاتصال هي المستوى الاول تردد صدى مجانا و _0،1 ≥ 180 كيلو هرتز وعامل جو...

Materials

Name	Company	Catalog Number	Comments
AFM XE-100	Park Instruments	discontinued	Atomic force microscope
CDT-NCLR	NanoSensors	CDT-NCLR	Conductive diamond coated non-contact lever
100 nm thick Au(111) thin film on Mica	Phasis	20020011	atomically smooth gold thin film