Medição de dureza quantitativa por Instrumented AFM-recuo

Resumo

This experimental protocol describes how atomic force microscopy can be used to measure hardness at the true nanometer scale and to detect single atomistic plasticity events.

Resumo

In this work, a combination of amplitude-modulated non-contact atomic force microscopy and atomic force spectroscopy is applied for instrumented hardness measurements on an Au(111) surface with atomistic resolution of single plasticity events. A careful experimental procedure is described that includes the force sensor selection, its calibration, the calibration of the cantilever deflection detection system, and the minimization of instrumental drift for accurate and reproducible force-distance measurements. Also, a method for the data analysis is presented that allows the extraction of force-penetration curves from recorded force-distance curves. A typical curve displays a clear elastic deformation regime up to the first plasticity event, or pop-in, with a length in the range of one to two Burger's vectors. Later plasticity events exhibit the same magnitude. The work of plasticity is further extracted from the measurements. Finally, the hardness is determined in combination with the indentation curve using non-contact atomic force microscopy images of the remaining indents.

Introdução

For the last 150 years, hardness values have been used to characterize the mechanical behavior of materials and to predict their performance under various loading conditions. The hardness of a material describes the resistance of its surface to the penetration of a harder indenter. For metallic materials, the hardness relates to resistance to plastic flow.

Although the implementation of hardness testing has proven to be relatively easy, the yielded results have long been rather empirical, making them unable to describe the intrinsic properties of the investigated material. The empirical nature of hardness testing results has been a consequence of the use of various indenter geometries, each of which has a different established hardness scale. However, all empirical hardness scales, such as the Brinell hardness (HB) test for spherical indenters and the Vickers (HV) and Knoop (HK) hardness tests for different types of four-sided pyramids, have one thing in common: the hardness number is determined from the ratio of the applied load to the developed area of the remaining indent. In an attempt to relate the hardness of metals to their fundamental mechanical properties, the hardness measured with a spherical indenter has been defined as the ratio of the applied load to the projected area of the remaining indent. This hardness value, also known as the Meyer's hardness (H), is equal to the mean contact pressure and directly relates to the yield strength of non-work-hardening metals¹.

Hardness testing has long been limited to the macro-scale, in which case the sizes of indents have been measured by optical means. The development of instrumented indentation, where force-displacement curves are recorded, has been recognized as a valuable alternative for determining the hardness of a material, although the size of the remaining indent may be too small to be accurately imaged. In this case, the projected area of the indent is calculated from the tip displacement according to a so-called indenter-area function². Due to this development, the analysis of the curvature of force-displacement curves and of the occurrence of distinct plasticity events in the shape of pop-ins is now widely used to study the mechanisms of plastic deformation at the micrometer scale, such as by means of nanoindentation³.

It is well accepted that the carriers of plastic deformation in metals, i.e., dislocations, operate at the nanometer scale. To understand their modes of operation at the atomic level, new experimental techniques with atomic resolution are needed. Initial investigations of atomistic plasticity events at the interfaces between nanometer-sized single asperities and single crystalline Au surfaces of different orientations have been carried out by interfacial force microscopy (IFM)^{4, 5}. Atomic force microscopy (AFM) indentation has been applied to observe the nucleation and gliding of single dislocations in KBr(100) single crystals⁶, Cu(100)⁷, and Au(111)^{8, 9}. There, atomistic plasticity events were observed in the shape of pop-ins, with lengths in the range of 1 Å. AFM indentation has also been used to measure the nano-hardness and -elasticity of gold nano-island grown on mica¹⁰. In this work, the nano-hardness was found to be smaller than the bulk value and was observed to depend linearly on the indentation area. Also, a detailed measurement protocol has been proposed to determine the hardness of hard surfaces, such as fused quartz and silicon, by AFM indentation with a diamond-tipped sapphire cantilever¹¹. In particular, this method takes into account the non-linearity of the photo-sensitive deflection detectors for large cantilever deflection¹². More recently, AFM indentation and non-contact AFM imaging have been combined to quantitatively determine the hardness and the fundamental mechanisms of plastic deformation of a Pt(111) single crystalline surface and Pt-based metallic glass¹³. For Pt(111), plastic deformation mechanisms at the nanometer scale were found to be consistent with the discrete mechanisms established for larger scales. Further, the nanometer-scale plastic deformation of the metallic glass was found to be not discrete, but rather continuous and highly localized around the indenting tip. These results revealed a lower size limit for metallic glasses, below which shear transformation mechanisms are not activated by indentation. AFM indentation has also been used to determine the hardness values of biological samples (such as collagen fibrils)¹⁴, polymers¹⁵, and colloidal crystals¹⁶.

In the present work, a careful experimental procedure is described that includes the force sensor selection, its calibration, the calibration of the cantilever deflection detection system, and the minimization of instrumental drift for accurate and reproducible force-distance measurements. Also, a method for the data analysis is presented that allows the extraction of force-penetration curves from recorded force-distance curves. Further representative results are shown and discussed in the light of recent findings in the field of plastic deformation of small volumes.

For this experiment, an atomic force microscope (AFM) was used. The cornerstone of an AFM is its micro-fabricated force sensor (usually a cantilever beam), with a sharp tip at the end whose radius is in the range of several nanometers. In the particular configuration of the instrument used for this experiment, the cantilever is mounted onto a piezo-electric z-scanner, while the sample to be investigated is mounted onto a piezo-electric x/y-scanner. During AFM imaging, the force sensor tip is scanned over a sample to register interaction forces with the sample surface that result in a deflection of the cantilever according to Hooke's law. The static or dynamic deflection of the cantilever can be measured with an optical beam deflection detector, consisting of a laser diode, a set of mirrors, and a photodiode that converts the cantilever deflection into a voltage. During imaging, the signal at the photodiode is controlled by a feedback loop so as to keep the interaction forces at the sample surface; this results in adjustments of the z-scanner position, which are recorded and displayed as a topography image.

Prerequisites for the experiment described below are that the piezo-electric scanners of the atomic force microscope are well-calibrated and that the instrument stays in the laboratory at a constant temperature and humidity level. The reader should be aware that, depending on the atomic force microscope model, some of the experimental procedure steps may have to be modified. In particular, all measurements are performed after setting the scanners' ranges to "small"; this function allows for the reduction of the x/y-scanner range to 5 x 5 µm² and the z-scanner range to 4 µm, which ensures a resolution at small scales. This function is not available on all commercial AFM and is not mentioned in the remainder of the text.

For data analysis, the use of the free SPM data analysis software Gwyddion¹⁷ and the Matlab software package¹⁸ are recommended.

This protocol gives a description of the experimental procedure to be followed in order to perform instrumented hardness measurements by AFM. Since the handling of different commercial AFMs may differ from one model to the other, the reader should refer to the manual provided by the AFM manufacturer for detailed setting procedures and software information. In the following text, in order to reproduce the described experiment, it is assumed that the reader is familiar with the handling of the particular AFM used here.

Protocolo

1. Instrumental Set-up e Calibração

1. Instrumental set-up
   1. Use um cantilever diamantada rígida do tipo DT-NCLR ou CDT-NCLR com uma primeira frequência de ressonância livre f _0,1 ≥ 180 kHz, um fator de qualidade Q ≥ 300, e uma rigidez de flexão k ≥ 40 N / m.
   2. Monte o cantilever selecionado em um suporte de fixação fornecido pelo fabricante do AFM. Tome especial cuidado para colocar o braço de suporte de tal modo que o seu eixo longitudinal é perpendicular à direcção de exploração rápida da AFM. Em alternativa, a cola cantilever sobre um suporte em consola fornecida pelo fabricante AFM utilizando cola epóxi de dois componentes.
   3. Montar o suporte do cantilever na cabeça AFM e usar o microscópio óptico normalmente disponíveis com o sistema AFM para focar o cantilever AFM. Verifique que longo eixo do cantilever é perpendicular à direção da digitalização rápido. Se não, voltar aSeção 1.1.2.
   4. Alinhar o feixe laser de modo a que se reflecte na extremidade do braço de suporte. Monitorar a soma de tensão no fotodíodo e realizar um ajuste fino para maximizar o sinal de soma. valores de sinal de soma típicas estão na gama de 2 V.
   5. Ajustar horizontais e verticais do ângulo de inclinação do espelho, de modo a trazer o ponto de laser reflectido para o centro do fotodíodo, onde as tensões correspondentes para o deslocamento vertical e lateral são quase zero.
2. calibragem
   1. Executar uma varredura de freqüência para determinar a primeira ressonância livre flexão f _0,1 do cantilever.
   2. Determinar a rigidez de flexão da consola K, calculadas de acordo com o ¹⁹
      (1)
      onde E é o módulo de Young, L é o comprimento do braço de suporte, W é a largura da cantilcada vez, e t é a sua espessura. Para este fim, medir o comprimento ea largura do cantilever por microscopia óptica ou microscopia eletrônica de varredura para maior precisão. Calcula-se a espessura do braço de suporte a partir da sua primeira frequência de ressonância livre flexão f _0,1, de acordo com a
      (2)
      onde ρ é a densidade em massa.
   3. Selecione o valor padrão da sensibilidade fotodiodo para o tipo cantilever particular a ser utilizado para o experimento no menu de configuração da AFM. Coloque a extremidade do cantilever em contacto com a amostra de referência com uma carga F _n = 10 nN clicando no botão abordagem.
   4. Abra o menu de espectroscopia de força no software AFM e definir a retração relativa e extensão do z-scanner para 50 nm e o z-scanner de retração / extensão a 0,3 mm / s. Fazendo isso, a gravação da curva força-distância seráconsistem em primeiro lugar de uma retracção da Z-digitalizador de 50 nm de distância da superfície da amostra e, em seguida, de uma série de abordagens e retracções da mesma distância.
   5. Gravar uma curva de força a distância com os parâmetros estabelecidos sugeridas no 1.2.4 sobre uma superfície lisa e não-compatível, como diamante nano-cristalino ou safira, a fim de evitar efeitos de deformação da amostra. Para fazer isso, clique no botão adquirir no menu de espectroscopia de força do software AFM.
   6. Coloque a peça repulsiva da curva força-distância com uma função linear, no menu de calibração do software AFM. A inclinação inversa da linha de montagem corresponde à sensibilidade fotodiodo S. Substitua o valor determinado para o valor padrão do software do aparelho no menu de calibração do software AFM, clicando no botão executar calibração.

Preparação 2. Amostra

NOTA: A amostra medido em this experiência consiste em um 100-nm de espessura atomicamente lisa de película fina, Au (111) cultivadas em mica por deposição física de vapor.

1. Montagem da amostra sobre um suporte de amostra magnética fornecida pelo fabricante do instrumento, por meio de fita de face dupla carbono. A fim de evitar o desvio da amostra durante a medição, a amostra montar um dia antes das medições, de forma a permitir que a fita de carbono relaxar. Alternativamente, a montagem da amostra sobre o suporte, com pintura de prata, que normalmente seca dentro de alguns minutos.
2. Montar o suporte de amostra magnética no scanner de x / y.

3. Processo de Medição

1. Defina a frequência de oscilação ligeiramente fora de ressonância (neste experimento f = 190,67 kHz) ea amplitude de oscilação em A = 20 nm Note-se que estes valores são definidos automaticamente pelo software do aparelho para este cantilever particular. Defina o ponto de ajuste de oscilação manualmente em um _set-point = 5 nm.
2. Desenharo braço de suporte na direcção da superfície da amostra, utilizando o motor passo a passo da AFM. Certifique-se de que o sensor de força não colidem com a superfície da amostra. Mantenha o cantilever em foco durante a aproximação grosseira e parar a abordagem grosseira antes de superfície da amostra está em foco perfeito.
3. Aproximar-se automaticamente o sensor de força, clicando no botão abordagem. Uma vez que a amplitude de oscilação atingiu o seu ponto de ajuste, a ponta está pronto para digitalizar a topografia da superfície da amostra.
4. Grave uma série de imagens de topografia em áreas que variam de 5 x 5 a 1 x 1 μm² (se disponível, ajustar a inclinação do sinal de topografia inclinando o x / y-scanner). Certifique-se de que as imagens sucessivas da mesma área não apresentam qualquer sinal de desvio e que a posição z do scanner permanece quase constante. Se este não for o caso, continuar a imagem até que o sistema estabilizou.
5. Uma vez que o sistema tenha se estabilizado e uma suave área μm² 1 x 1 foi encontrado, retrair o force sensores alguns micrômetros de superfície da amostra clicando no botão de retorno.
6. Seleccionar o modo de espectroscopia de força no menu de instrumentos e mover o sensor de força para o meio da pré-seleccionada área μm² 1 x 1, com um ponto de ajuste da força de 10 nm. Monitorar a posição do z-digitalizador até que esta se mantém constante.
7. Escolha da grelha de 2 x 2 de pontos cujo centro corresponde ao centro da área pré-seleccionada μm² 1 x 1. Defina a distância entre dois pontos vizinhos próximos a 500 nm.
8. Definir a distância em relação ao digitalizador variar de 0 a 150 nm, a uma velocidade de 300 nm / s e, em seguida, a retrair ao longo da mesma distância e na mesma velocidade. Dado o ângulo de inclinação do cantilever em relação à superfície da amostra, aplica uma correcção de inclinação, movendo o scanner laterais por Z x tan φ durante uma extensão do scanner Z vertical, onde φ é o ângulo de inclinação ^20.
   NOTA: Alguns instruments representam a inclinação cantilever em sua espectroscopia de força ou o modo de recuo; este é o caso para a AFM utilizado neste trabalho.
9. Pressione o botão Iniciar no software do aparelho para iniciar a aquisição dos dados AFM recuo.
10. Uma vez que as medidas de AFM de recuo ter sido concluída, retrair o sensor de força de alguns micrómetros de distância da superfície da amostra.
11. Selecione o não-contato de imagem modo AFM no menu de software do aparelho e repita o procedimento descrito nas secções 3.1 e 3.2.
12. Executar uma verificação sobre a mesma área de superfície 1 x 1 μm² como na Seção 3.3, de modo a localizar a posição exata dos travessões. Mais exames de superfície sobre um 500 x 500 área superficial nm² pode ser realizada para a imagem dos travessões restantes com maior detalhe.

Análise 4. Dados

1. Processamento de imagem
   1. Processar as imagens gravadas topografia de modo a alinhar as linhas no dir varredura rápidaexão com base na diferença média. Use a função built-in de Gwyddion.
2. Calcule a área projectada A _p de recuos usando a função de análise recuo de Gwyddion.
3. Estimar a forma da ponta AFM a partir das imagens de topografia de travessões usando a função de análise de ponta do Gwyddion. Em seguida, calcular a média das imagens de forma da ponta e medir o ângulo α da forma da ponta média de meia-abertura.
4. Converter as curvas força-distância em curvas força-deslocamento através do cálculo do deslocamento da ponta δ de acordo com a ¹³
   (3)
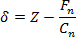
   onde Z é a posição relativa do scanner.
5. Agora, traçar a força versus o deslocamento da ponta. A curva resultante exibe geralmente chamados pop-ins, com comprimentos na gama de vários pm 100, que correspondem a eventos de plasticidade atomísticas. Use o primeiro de these pop-ins para determinar o deslocamento da ponta no limite elástico δ _el ^4.
6. Coloque a peça elástica da curva de força-deslocamento com a função hertziana ^21.
   (4)
   em que R é o raio da ponta e E ^'* é o módulo de elasticidade reduzido de, dada pela , Com o M _{S, T} sendo o módulo de recuo da amostra e da ponta, respectivamente. Neste caso, o parâmetro de ajuste é .
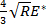7. Estender a função de ajuste no regime de plasticidade, de modo a calcular o trabalho da plasticidade W _plasticidade da diferença de área entre a função de ajuste ea curva experimental ^21.
8. Calcula-se a dureza da amostra de acordo com ^{um, dois}
   (5)
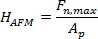
   e
   (6)
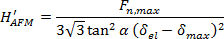
   onde F _{n, max} é a carga máxima aplicada, A _p é a área projetada do travessão calculado na Seção 4.2, α é o ângulo da ponta calculado na Seção 4.3 meia de abertura, δ _el é o deslocamento da ponta na primeira plasticidade evento, e δ _max é o deslocamento da ponta máxima (ver secção 4.4).

Resultados

Neste trabalho, a rigidez de flexão do cantilever K foi calculado de acordo com a teoria de feixe ¹⁹ geométrica. Para o particular cantilever revestido de diamante utilizado neste trabalho, encontramos k = 55,69 N / m. Note que nós negligenciado o revestimento de diamante; a espessura do revestimento de diamante é de uma a duas ordens de grandeza menor do que a espessura do braço de suporte e, assim, não aumenta significativamente a sua rigidez à flex?...

Discussão

Um método tem sido apresentado para a realização de uma série de recortes em uma Au (111) da superfície de película fina com uma ponta de AFM de diamante-revestido. Sem contacto de imagem AFM e recuo AFM foram realizados com o mesmo sensor de força. Os requisitos para a imagem sem contato são uma primeira frequência de ressonância de alta f _0,1 ≥ 180 kHz e um fator de alta qualidade Q ≥ 300. Em AFM recuo, a força vertical a ser aplicada é na faixa de vários micro-newtons, e ...

Materiais

Name	Company	Catalog Number	Comments
AFM XE-100	Park Instruments	discontinued	Atomic force microscope
CDT-NCLR	NanoSensors	CDT-NCLR	Conductive diamond coated non-contact lever
100 nm thick Au(111) thin film on Mica	Phasis	20020011	atomically smooth gold thin film