Hot Biological Catalysis: Isothermal Titration Calorimetry to Characterize Enzymatic Reactions

Summary

Isothermal titration calorimetry measures heat flow released or absorbed in chemical reactions. This method can be used to quantify enzyme-catalysis. In this paper, the protocol for instrumental setup, experiment running, and data analysis is generally described, and applied to the characterization of enzymatic urea hydrolysis by jack bean urease.

Abstract

Isothermal titration calorimetry (ITC) is a well-described technique that measures the heat released or absorbed during a chemical reaction, using it as an intrinsic probe to characterize virtually every chemical process. Nowadays, this technique is extensively applied to determine thermodynamic parameters of biomolecular binding equilibria. In addition, ITC has been demonstrated to be able of directly measuring kinetics and thermodynamic parameters (k_cat, K_M, ΔH) of enzymatic reactions, even though this application is still underexploited. As heat changes spontaneously occur during enzymatic catalysis, ITC does not require any modification or labeling of the system under analysis and can be performed in solution. Moreover, the method needs little amount of material. These properties make ITC an invaluable, powerful and unique tool to study enzyme kinetics in several applications, such as, for example, drug discovery.

In this work an experimental ITC-based method to quantify kinetics and thermodynamics of enzymatic reactions is thoroughly described. This method is applied to determine k_cat and K_M of the enzymatic hydrolysis of urea by Canavalia ensiformis (jack bean) urease. Calculation of intrinsic molar enthalpy (ΔH_int) of the reaction is performed. The values thus obtained are consistent with previous data reported in literature, demonstrating the reliability of the methodology.

Introduction

Quantitative determination of biochemical reactions provides insights into the biological processes at the basis of life. Calorimetry offers a label-free methodology to quantitatively characterize virtually every chemical reaction in solution. This technique measures the heat released or absorbed over time, and is therefore a universal detection system and a very convenient methodology to quantify the amount of reacting molecules (i.e. binding thermodynamics), as well as to measure the reaction rate (i.e. kinetics). In particular, isothermal titration calorimetry (ITC) has been adopted as method of choice to characterize the thermodynamics of biomolecular equilibria, involving protein-ligand, protein-protein, protein-metal ions and protein-DNA interactions^1-6. In addition, the ability of ITC to provide kinetic information makes it a very powerful system to measure enzyme catalysis, although the potential of this application is still underestimated^7-9.

The Michaelis-Menten equation¹⁰ is a quantitative description of enzymatic reactions, as it provides a relationship between the reaction rate and the substrate concentration, depending on two kinetic parameters: the Michaelis constant (K_M) and the catalytic rate constant (k_cat). The k_cat/K_M ratio is referred as the catalytic efficiency of an enzyme. In practice, determination of K_M and k_cat for a specific reaction provides a complete description of the catalysis.

In a typical enzymatic reaction (Figure 1), a substrate (S) interacts with the enzyme (E) forming the enzyme-substrate (ES) complex, which is subsequently activated into the transition state (ES*). The latter is converted into the enzyme-product (EP) complex that eventually dissociates. These steps are described by the following reaction.

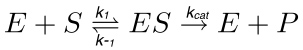(1)

where k₁ is the rate constant for the formation of the ES complex, k_-1 is the rate constant for the dissociation of the ES complex, while k_cat is the catalytic rate constant or turnover number.

According to the Michaelis-Menten equation¹⁰, the rate of the reaction can be calculated as:

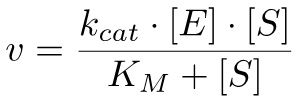(2)

in which K_M = (k_-1 + k_cat)/k₁ and k_cat = v_max/[E], with v_max being the maximal velocity reached when all enzyme is bound to the substrate.

The isothermal titration calorimeter is the instrument used in this study to characterize the enzymatic hydrolysis of urea. This instrument is made of an adiabatic shield containing two coined-shaped cells (Figure 1). These are connected to the outside with narrow access tubes. The sample cell (ca. 1.4 ml) is loaded with the enzyme solution, while the reference cell is generally filled with water or with the solvent used for the analysis. A rotating syringe with a long needle and a stir paddle attached, usually containing ca. 0.3 ml of substrate solution, is mounted on the sample cell. A thermoelectric device measures the difference of temperature between the sample and the reference cell and, using a “cell feedback network”, it maintains this difference at zero by adding or subtracting heat. During the experiment, the substrate is injected into the enzyme solution at a constant chosen temperature. When the enzymatic reaction takes place, the amount of heat released or absorbed is proportional to the number of substrate molecules that are converted into product molecules. In addition, the rate of heat flow is directly related to the rate of the reaction. The measured data, appearing as a deviation of the heat trace from initial baseline (Figure 1), represent the thermal power (μcal/sec) supplied by the instrument to the sample cell, which is proportional to the heat flow occurring in the sample cell over time.

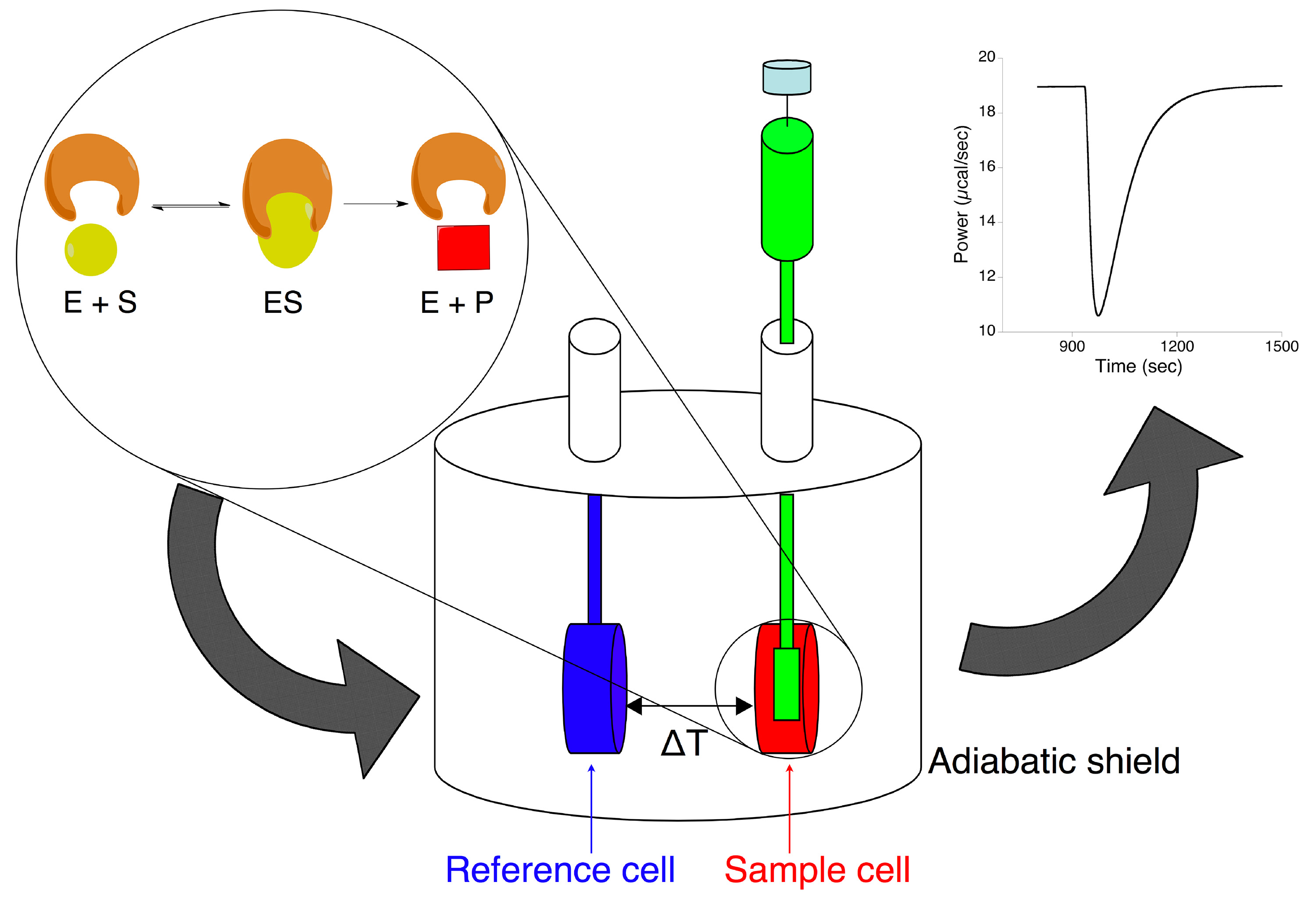
Figure 1. Schematic representation of isothermal titration calorimeter to study enzymatic reactions. An enzymatic reaction occurring upon titration of the substrate (in the injection syringe) into the enzyme solution (in the sample cell) results in a change of the thermal power released by the calorimeter, needed to keep the difference of temperature between the sample cell and the reference cell constant. Click here to view larger image.

Overall, the heat change (Q) is proportional to the molar enthalpy of the reaction (ΔH) and the number of moles of product generated (n), which in turn is given by the total volume times the concentration:

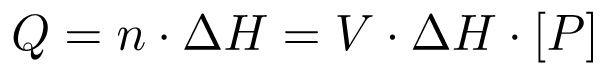(3)

The product formation over time (dP/dt), which corresponds to the reaction rate, can thus be related to the amount of heat generated over the same time (dQ/dt) through the relation:

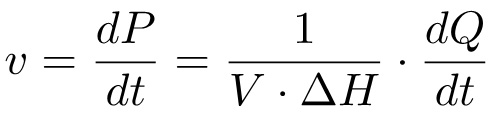(4)

According to this equation, in order to obtain a Michaelis-Menten plot it is necessary to measure i) the total molar enthalpy ΔH, and ii) the heat flow dQ/dt at different substrate concentrations. Usually, this is performed in two different experiments: in the first experiment (Method 1, M1), the substrate is injected into the enzyme solution and the heat for complete substrate conversion is measured; in the second experiment (Method 2, M2), multiple injections of substrate are performed and the rate of heat production is measured at different substrate concentrations. These two sets of data are sufficient to derive the kinetic parameters K_M and k_cat.

In the present article, a general protocol to determine the kinetic parameters for enzymatic reactions performed using ITC is described. We applied the method to urea hydrolysis by Canavalia ensiformis urease, as a reference system. The good agreement between the results obtained using this method and the data reported in literature demonstrates the reliability of this approach.

Protocol

1. Preparing Samples

1. Prepare 2 ml of enzyme solution and 0.5 ml of substrate solution for each experimental run. Dilute concentrated stock solutions of enzyme and substrate in buffer solutions having identical composition to minimize the heat of dilution and mixing during the substrate addition.
   1. Choose the buffer conditions that are adequate to prevent pH change during experiment. For example, 20 mM HEPES pH 7 is adequate for measurements at neutral pH.
      NOTE: If proton exchange is involved, protonation enthalpies of the used buffers must be considered, because they affect the measured ΔH of the reaction. Possible specific effects of the buffer or additives molecules on the system under analysis must be taken into account. If organic solvents (e.g. DMSO) are included in the enzyme solution, add them at exactly the same concentration into the substrate solution.
   2. For the M1 experiment, start with enzyme concentration in the nM range (e.g. 1 nM) and with a substrate concentration at least three orders of magnitude higher than the enzyme concentration and above the K_M.
   3. For the M2 experiment, start with enzyme concentration in the pM-nM range (e.g. 15 pM). Substrate concentration in the syringe is in the mM range (e.g. 400 mM).
      NOTE: In the single injection M1 experiment, concentrations should be adequate to achieve the total substrate conversion into the product over the experimental time. Therefore, the concentration of the enzyme depends on the enzyme rate: if enzymes with low k_cat are under study, higher enzyme concentrations (up to 10 µM) must be used. On the other hand, enzyme concentrations used in the M2 experiment must assure that the injected substrate is only marginally (less than 5%) consumed and that the enzyme reaction proceeds at the steady state. For this reason, the higher the enzyme efficiency, the lower the required enzyme concentration. At the end of the experiment, substrate concentration in the sample cell should be higher than K_M.
2. Carefully check enzyme and substrate concentrations with an appropriate analytical procedure (e.g. absorbance at 280 nm, colorimetric, BCA assays¹¹. This is required to obtain accurate and reliable calculation of the thermodynamic and kinetic parameters.
3. Measure the pH of the solutions and verify that the pH mismatch of both the enzyme and the substrate solutions is minimal under the experimental conditions (±0.05 pH units).

2. Performing the Experiment

NOTE: The same procedure must be applied both for the M1 and the M2 experiment, which are performed one after the other.

1. Verify that the sample cell and the injection syringe are cleaned according to the manufacturer’s instructions. Fill the loading syringe provided with the instrument with distilled water, gently insert the needle in the sample cell, fill the cell and remove the liquid using the same syringe. Using this method, wash the sample cell twice with distilled water and twice with the buffer.
2. Load the sample cell with 2 ml of enzyme solution using the loading syringe, carefully avoiding formation of air bubbles. Slowly inject the solution into the cell until it spills out the top of the cell stem. Produce a spurt of ca. 0.25 ml of solution into the cell. Repeat twice. This step removes air bubbles trapped in the sample cell.
3. Place the needle of the loading syringe on the ledge between the cell stem and the cell port and remove any excess solution.
4. Start the VP-Viewer program and, from the computer interface, equilibrate the ITC instrument to a temperature 3 °C below the desired experimental temperature. This is required to avoid long equilibration periods before the experiment, due to the passive instrumental cooling device.
5. Fill the reference cell with distilled water with the same procedure above. When buffers with high ionic strength or high osmolality are in the sample solution, the same buffer should be used as a reference solution.
6. Link a plastic syringe to the fill port of the injection syringe, using a thin silicon tube. Fill the injection syringe placing the needle tip into water and drawing up, until water comes out of the top filling port, indicating that the injection syringe is full. Then move the syringe tip from water and draw up air, to empty the injection syringe. With this procedure, wash the injection syringe with buffer, and draw air through the system. Subsequently, place the injection needle in a narrow tube containing 0.5 ml of substrate solution, carefully draw up and completely fill the injection syringe, leaving small amount of solution at the bottom of the tube.
7. From the computer interface, press “Close fill port”. Remove the silicon loading tube. Press “Purge and refill” button to allow the injection syringe to dislodge any air bubbles and to expel them back into the bulk solution. Repeat twice.
8. Move the injection syringe, wipe on the sides to remove any drops and place the needle of the injection syringe into the sample cell.
9. On the computer, set the appropriate running parameters. The experimental enzyme and substrate concentrations can be indicated.
   1. In the M1 experiment, set at least two additions (5-30 µl) of substrate to verify the reproducibility. Set the spacing time between each injection (e.g. 1,000 sec) large enough to ensure that the heat signal returns to the baseline before the next addition.
   2. In the M2 experiment, set multiple (e.g. 15 x 5-10 µl) injections. Set the interval between the injections (e.g. 180 sec) allowing the system to stabilize the thermal power to the new baseline after each injection.
      NOTE: The spacing time between injections in the M2 experiment should be short enough to avoid the conversion of a significant amount of substrate, allowing the measurements to be performed under steady state conditions.
   3. In the M2 experiment, use small volumes (e.g. 2 μl) for the first injection, whose corresponding value is discarded during the subsequent data analysis. Indeed, it often presents artifacts due to the initial substrate diffusion through the syringe tip, and for the presence of air bubbles trapped in the syringe needle.
   4. Set the reference power, the approximate level in which the baseline will be placed before the reaction starts, at a value of 20. Then define the experimental enzyme and substrate concentrations and chose a name for the experiment.
   5. Define the experimental temperature, typically at 25 °C. ITC allows working temperatures between 2 °C and 80 °C. The experiment is ready for running. Press “Start” button to start the experiment.
10. Once the experiment has finished, clean the sample cell and the syringe according to the manufacturer's instructions.
11. Repeat the experiment at least one or two more times to check the reproducibility of the data.

3. Data Analysis

1. From the analysis program, click on the “Read data” button, and navigate to the folder where the .itc file of the performed M2 experiment is located. Click on the scroll down arrow of the “Files of type” and select “Enzyme Assay (it?)”. Subsequently, click and open the .itc file of the M2 experiment.
2. Obtain the ΔH of the reaction from integrating the curve of the M1 experiment according to Equation 5.
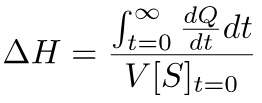   (5)
   1. Click on the “Read data” button, and select the file .itc of the performed M1 experiment. Using Origin, divide the trace in different parts containing one peak each and save each peak as an .opj file. Open the file corresponding to the first peak, resulting from baseline deviation in the thermogram of the single injection M1 experiment, integrate it and divide the obtained area value, expressed in µcal, by the final substrate concentration in the sample cell, expressed in µM, and by the cell volume expressed in liters, determining, according to Equation 5, the ΔH of the reaction.
   2. Repeat the same procedure for the second peak of the M1 experiment and obtain an average value for the two ΔH measurements.
3. Determine dQ/dt from the M2 experiment measuring the difference between the original baseline and the new baseline following each injection. Convert the experimental data to reaction rates according to Equation 4, using the enthalpy value obtained in the M1 experiment and fit the data to the Michaelis-Menten equation.
   1. From the analysis program, click on the “Read data” button, and select the file .itc of the performed M2 experiment. Click on the scroll down arrow of the “Files of type” and select “Enzyme Assay (it?)”.
   2. The Enzyme Assay dialog box opens, allowing selecting one of the four models. Select “Method 2 - Substrate only” from the window.
   3. In the ΔH window, indicate the value of ΔH obtained in step 4.2.
   4. Click the “Concentration” button to check the concentration values to be used in the fitting procedure. Click the “Average Time (P)” button. The dialog box opens giving the opportunity to change or accept the default value. This value represents the time before each injection in which the instrument averages the power signal to determine the power level at each substrate concentration. Click OK to confirm the default value.
   5. Click the “Zero Y Axis” button. The cursor turns to a cross hair allowing to double click a point, to place at y=0. Double click just before the first injection point.
   6. Click the Calculate Rate button. Rate is plotted versus the substrate concentration, derived by dividing the substrate added during the titration for the sample cell volume (taking into account dilution effects). This operation gives a typical Michaelis-Menten plot that can be fitted to obtain K_M and k_cat.
   7. If some points should be deleted, select the “Truncate Data” button. Move the data markers to exclude the bad data points and double click on one of the markers or press enter.
   8. Use the “Fit to model” function to fit the curve and to obtain the kinetic constants.

Results

Urease (EC 3.5.1.5; urea amidohydrolase) is a multisubunit nickel-containing enzyme found in archea, bacteria, unicellular eukaryotes and plants. This protein acts in the last step of organic nitrogen mineralization, catalyzing the hydrolysis of urea to ammonia and carbamate, which spontaneously decomposes to give a second molecule of ammonia and bicarbonate (Equation 6)¹².

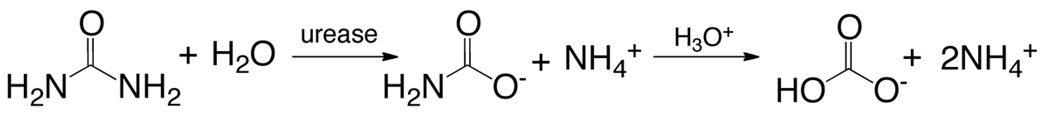(6)

...

Discussion

Significance of ITC to study enzymatic activity with respect to existing methods

In addition to its classical applications to study binding equilibria, isothermal titration calorimetry provides a reliable and fast method to characterize enzymatic reactions in solution using the heat of reaction as a probe, without requiring system modification or labeling. The kinetic parameters k_cat and K_M are usually obtained through a set of time cour...

Materials

Name	Company	Catalog Number	Comments
HEPES	Sigma	H3375	dissolving in water and adjusting pH with NaOH
TRIZMA-Base	Sigma	T1503	dissolving in water and adjusting pH with HCl
Sodium dihydrogen phosphate	Riedel-de-Haen	4270	dissolving in water
Sodium phosphate dibasic	Riedel-de-Haen	30427	dissolving in water
Urea	Sigma	U4128	dissolving in water at 40 °C
Canavalia ensiformis urease (type C-3)	Sigma	U0251	dissolving in 20 mM HEPES pH 7 and stored at -80 °C
VP-ITC on Origin 7.0	MicroCal (GE Healthcare)	SYS13901	instrument
VPViewer2000 1.30.00 on Origin 7.0	MicroCal (GE Healthcare)		data acquisition software supplied with the instrument